Page 303 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes 1977 5 18.7 19.6

1978 6 18.6 20.3
1979 7 21.5 21.0

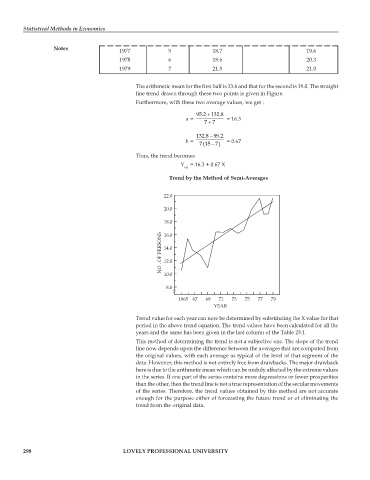
The arithmetic mean for the first half is 13.6 and that for the second is 19.0. The straight
line trend drawn through these two points is given in Figure.
Furthermore, with these two average values, we get :

95.2 132.8
+
a = = 16.3
+
77
−
132.8 95.2
b = ( − ) 715 7 = 0.67
Thus, the trend becomes
Y = 16.3 + 0.67 X
cal
Trend by the Method of Semi-Averages

22.0

20.0
18.0

PERSONS 16.0

14.0
OF . 12.0
NO
10.0

8.0
1965 67 69 71 73 75 77 79
YEAR
Trend value for each year can now be determined by substituting the X value for that
period in the above trend equation. The trend values have been calculated for all the
years and the same has been given in the last column of the Table 23.1.
This method of determining the trend is not a subjective one. The slope of the trend
line now depends upon the difference between the averages that are computed from
the original values, with each average as typical of the level of that segment of the
data. However, this method is not entirely free from drawbacks. The major drawback
here is due to the arithmetic mean which can be unduly affected by the extreme values
in the series. If one part of the series contains more depressions or fewer prosperities
than the other, then the trend line is not a true representation of the secular movements
of the series. Therefore, the trend values obtained by this method are not accurate
enough for the purpose either of forecasting the future trend or of eliminating the
trend from the original data.

298 LOVELY PROFESSIONAL UNIVERSITY