Page 340 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 26: Theory of Probability: Introduction and Uses

Notes
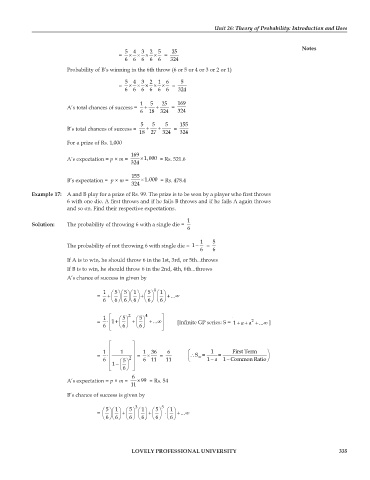
5 4 3 2 5 25
= ×× ×× =
6 6 6 6 6 324
Probability of B’s winning in the 6th throw (6 or 5 or 4 or 3 or 2 or 1)
5 4 3 2 1 6 5
= × × × × × =
6 6 6 6 6 6 324

1 5 25 169
A’s total chances of success = + + =
6 18 324 324
5 5 5 155
B’s total chances of success = + + =
18 27 324 324
For a prize of Rs. 1,000
169
A’s expectation = p × m = × 1,000 = Rs. 521.6
324

155
B’s expectation = p × m = × 1,000 = Rs. 478.4
324
Example 17: A and B play for a prize of Rs. 99. The prize is to be won by a player who first throws
6 with one die. A first throws and if he fails B throws and if he fails A again throws
and so on. Find their respective expectations.

1
Solution: The probability of throwing 6 with a single die =
6
1 5
The probability of not throwing 6 with single die = −1 =
6 6
If A is to win, he should throw 6 in the 1st, 3rd, or 5th...throws
If B is to win, he should throw 6 in the 2nd, 4th, 6th...throws
A’s chance of success in given by
4
5
5
1
1
5
1 ⎛ ⎞⎛⎞⎛⎞ ⎛⎞ ⎛⎞
+
= + ⎜ ⎟⎜⎟⎜⎟ ⎜⎟ ⎜⎟ + ... ∞
6
6 ⎝ ⎠⎝⎠⎝⎠ ⎝⎠ ⎝⎠
6
6
6
6
5
5
1 ⎡ ⎛⎞ 2 ⎛⎞ 4 ⎤
= ⋅ ⎢ + ⎜⎟ + ⎜⎟ + ... ∞ ⎥1 [Infinite GP series: S = ++1 aa 2 +... ∞ ]
6 ⎢ ⎝⎠ ⎝⎠ ⎥ ⎣ ⎦
6
6
⎡ ⎤
1 ⎢ ⎢ 1 ⎥ ⎥ 1 36 6 ⎛ 1 First Term ⎞
= 6 ⎢ ⋅ 5 2 ⎥ = 6 × 11 = 11 ⎜ ⎝ ∴S= 1 − = 1 Common Ratio ⎟ ⎠
∞
− a
⎛⎞
⎢ 1 − ⎜⎟ ⎥
⎣ ⎝⎠ ⎦ 6
6
A’s expectation = p × m = × 99 = Rs. 54
11
B’s chance of success is given by
3
1
⎛⎞⎛ ⎞ ⎛⎞ ⎛⎞ ⎛⎞ 5 ⎛⎞
5
1
5
5
1
+
+
= ⎜⎟⎜ ⎟ ⎜⎟ ⎜⎟ ⎜⎟ ⋅ ⎜⎟ + ... ∞
6
6
6
6
6
6
⎝⎠⎝ ⎠ ⎝⎠ ⎝⎠ ⎝⎠ ⎝⎠
LOVELY PROFESSIONAL UNIVERSITY 335