Page 45 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 4: Central Tendency: Mean, Median and Mode and their Properties

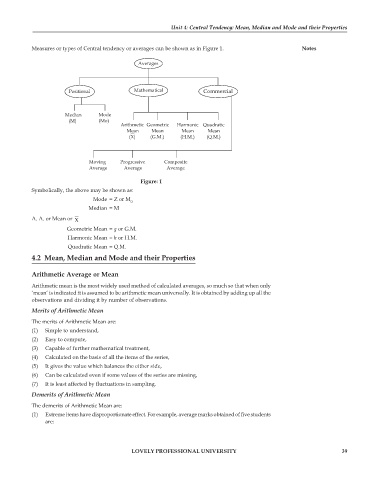
Measures or types of Central tendency or averages can be shown as in Figure 1. Notes

Averages

Positional Mathematical Commercial

Median Mode
(M) (Mo)
Arithmetic Geometric Harmonic Quadratic
Mean Mean Mean Mean
(X) (G.M.) (H.M.) (Q.M.)

Moving Progressive Composite
Average Average Average

Figure: 1
Symbolically, the above may be shown as:
Mode = Z or M
O
Median = M
A. A. or Mean or X
Geometric Mean = g or G.M.
Harmonic Mean = h or H.M.
Quadratic Mean = Q.M.
4.2 Mean, Median and Mode and their Properties

Arithmetic Average or Mean
Arithmetic mean is the most widely used method of calculated averages, so much so that when only
‘mean’ is indicated it is assumed to be arithmetic mean universally. It is obtained by adding up all the
observations and dividing it by number of observations.
Merits of Arithmetic Mean

The merits of Arithmetic Mean are:
(1) Simple to understand,
(2) Easy to compute,
(3) Capable of further mathematical treatment,
(4) Calculated on the basis of all the items of the series,
(5) It gives the value which balances the either side,
(6) Can be calculated even if some values of the series are missing,
(7) It is least affected by fluctuations in sampling.

Demerits of Arithmetic Mean
The demerits of Arithmetic Mean are:
(1) Extreme items have disproportionate effect. For example, average marks obtained of five students
are:

LOVELY PROFESSIONAL UNIVERSITY 39