Page 48 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes (3) It is not much affected by the extreme values in the series.
(4) Capable of being applicable to study social and economic phenomena.
Demerits

(1) If any value is ‘0’ (zero), G.M. cannot be calculated (because (X ), (X ) ... (X ) is to be found. If
1 2 n
any of these is zero, the multiplication result will be zero and interpretation would become
impossible.
(2) Knowledge of logarithms is essential. Therefore it is found difficult to compute by a non-
mathematics background person.
(3) Difficult to locate.
(4) G.M. cannot be calculated if even one value of the series is not available.
(5) The value of G.M. obtained may not be there in the series, therefore, it cannot be termed as the
true representative of the data.
Mathematical Properties of the Arithmetic Mean
The following are a few important mathematical properties of the arithmetic mean:
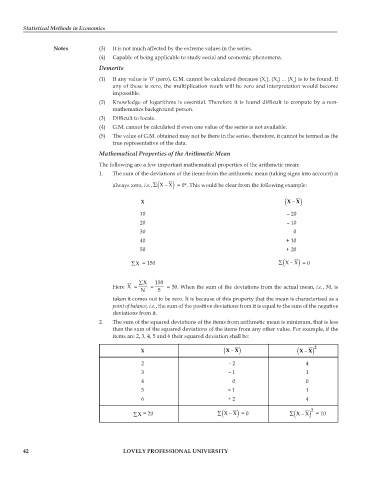
1. The sum of the deviations of the items from the arithmetic mean (taking signs into account) is
always zero, i.e., ( ∑ X = 0*. This would be clear from the following example:
) − X

X ( − X ) X
10 – 20
20 – 10
30 0
40 + 10
50 + 20

∑X = 150 ∑ ( X = 0
) − X

∑X 150
Here X = = = 30. When the sum of the deviations from the actual mean, i.e., 30, is
N 5
taken it comes out to be zero. It is because of this property that the mean is characterised as a
point of balance, i.e., the sum of the positive deviations from it is equal to the sum of the negative
deviations from it.
2. The sum of the squared deviations of the items from arithmetic mean is minimum, that is less
than the sum of the squared deviations of the items from any other value. For example, if the
items are 2, 3, 4, 5 and 6 their squared deviation shall be:

X ( − ) XX ( XX ) − 2
2 – 2 4
3 – 1 1
4 0 0
5 + 1 1
6 + 2 4

∑X = 20 ∑ ( − X = 0 ∑ ( ) − XX 2 = 10
) X

42 LOVELY PROFESSIONAL UNIVERSITY