Page 142 - DMTH401_REAL ANALYSIS

Real Analysis

Notes For, if lim f(x) = A, then for a given  > 0, there must exist some  > 0, such that |f(x) – A| < . Let
x 0
us choose x, > 0, x, < 0 such that |x | < S and |x | < . Then
1 2
2 = |f(x ) – f(x )|
1 2
 |f(x ) – A| + |A – f(x )|
1 2
< 2, (because |x – 0| <  and |x – 0| < )
1 2
for every  which is clearly impossible if  < 1. Non-existence of lim f(x) also follows from
x 0
Theorem 2, since f(0+)  f(0–).
The above example shows clearly that the existence of both f(a+) and f(a–) alone is not sufficient
for the existence of lim f(x). In fact, for lim f(x) to exist, they both should be equal.
x 0 x a
1
Now consider, the function f defined by f(x) = for x  0.
x
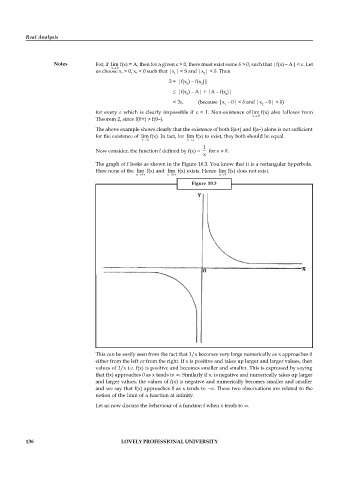
The graph of f looks as shown in the Figure 10.3. You know that it is a rectangular hyperbola.
Here none of the lim f(x) and lim f(x) exists. Hence lim f(x) does not exist.
0
x + x + x 0
0
Figure 10.3

This can be easily seen from the fact that 1/x becomes very large numerically as x approaches 0
either from the left or from the right. If x is positive and takes up larger and larger values, then
values of 1/x i.e. f(x) is positive and becomes smaller and smaller. This is expressed by saying
that f(x) approaches 0 as x tends to . Similarly if x, is negative and numerically takes up larger
and larger values, the values of f(x) is negative and numerically becomes smaller and smaller
and we say that f(x) approaches 0 as x tends to –. These two observations are related to the
notion of the limit of a function at infinity.
Let us now discuss the behaviour of a function f when x tends to .

136 LOVELY PROFESSIONAL UNIVERSITY