Page 150 - DMTH401_REAL ANALYSIS

Real Analysis

Notes
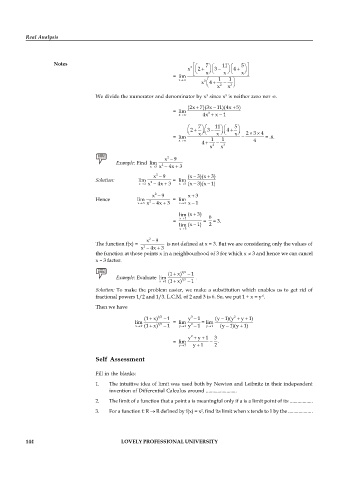
3 éæ 7 ö æ 11ö æ 5 ù ö
x ç ê 2 + ÷ ç 3 - ÷ ç 4 + ÷ ú
ë è x ø è x ø è x ø û
= lim
x 3 æ 1 1 ö
x ç 4 + - 3 ÷
è x 2 x ø
We divide the numerator and denominator by x since x is neither zero nor .
3
3
(2x 7)(3x 11)(4x 5)
+
-
+
= lim 3
x 4x + x - 1
æ 7 ö æ 11ö æ 5 ö
ç 2 + ÷ ç 3 - ÷ ç 4 + ÷
´
´
è x ø è x ø è x ø 2 3 4
= lim - = .6.
x 1 1 4
4 + -
x 2 x 3
2
x - 9
Example: Find lim
2
x 3 x - 4x + 3
2
-
+
x - 9 (x 3)(x 3)
Solution: lim 2 = lim
x 3 x - 4x + 3 x 3 (x 3)(x 1)- -
2
+
x - 9 x 3
Hence lim 2 = lim
x 3 x - 4x + 3 x 3 x 1-
+
lim (x 3)
x 3 6
= = = 3.
-
lim (x 1) 2
x 3
2
x - 9
The function f(x) = is not defined at x = 3. But we are considering only the values of
2
x - 4x + 3
the function at those points x in a neighbourhood of 3 for which x  3 and hence we can cancel
x – 3 factor.
(1 x) 1 2 - 1
+
Example: Evaluate lim 1 3 .
x 0 (1 x)+ - 1
Solution: To make the problem easier, we make a substitution which enables us to get rid of
fractional powers 1/2 and 1/3. L.C.M. of 2 and 3 is 6. So, we put 1 + x = y .
6
Then we have
2
3
-
+
(1 x) 1 2 - 1 y - 1 (y 1)(y + y 1)
+
lim 1 3 = lim 2 = lim
-
+
x 0 (1 x)+ - 1 y 1 y - 1 y 1 (y 1)(y 1)
2
+
y + y 1 3
= lim - .
+
y 1 y 1 2
Self Assessment
Fill in the blanks:
1. The intuitive idea of limit was used both by Newton and Leibnitz in their independent
invention of Differential Calculus around ........................
2. The limit of a function that a point a is meaningful only if a is a limit point of its ..................
2
3. For a function f: R  R defined by f(x) = x , find its limit when x tends to 1 by the .
144 LOVELY PROFESSIONAL UNIVERSITY