Page 189 - DMTH401_REAL ANALYSIS

Unit 14: Sequences and Series of Functions

Notes

1 1
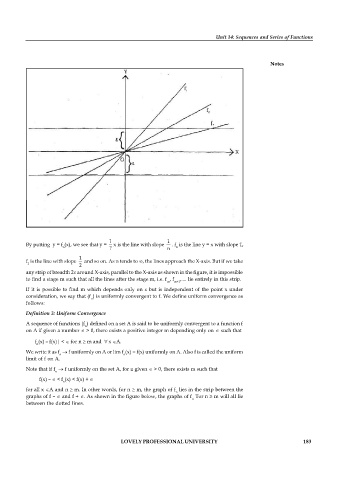
By putting y = f (x), we see that y = x is the line with slope . f is the line y = x with slope 1,
n ? n n
1
f is the line with slope and so on. As n tends to ¥, the lines approach the X-axis. But if we take
2
2
any strip of breadth 2 around X-axis, parallel to the X-axis as shown in the figure, it is impossible
to find a stage m such that all the lines after the stage m, i.e. f , f ,.... lie entirely in this strip.
m m+1
If it is possible to find m which depends only on  but is independent of the point x under
consideration, we say that (f ) is uniformly convergent to f. We define uniform convergence as
n
follows:
Definition 3: Uniform Convergence
A sequence of functions (f ) defined on a set A is said to be uniformly convergent to a function f
n
on A if given a number  > 0, there exists a positive integer m depending only on  such that
f (x) – f(x)| <  for n  m and " x A.
n
We write it as f ® f uniformly on A or lim f (x) = f(x) uniformly on A. Also f is called the uniform
n n
limit of f on A.
Note that if f ® f uniformly on the set A, for a given > 0, there exists m such that
n
f(x) –  < f (x) < f(x) + 
n
for all x A and n  m. In other words, for n  m, the graph of f lies in the strip between the
n
graphs of f– and f + . As shown in the figure below, the graphs of f Tor n  m will all lie
n
between the dotted lines.

LOVELY PROFESSIONAL UNIVERSITY 183