Page 326 - DMTH401_REAL ANALYSIS

Real Analysis

Notes By Proposition, each set on the right is measurable, thus so is (f) [a, ]. We’ll analyze more
–1
algebraic properties of measurable functions in the next section.
We now give some examples of measurable functions.

Example: Let X =  with Lebesgue measure. Then any continuous function f :    is
n
n
measurable because for any a  , by continuity (the inverse of any open set is open),
–1
–1
f [a, ] = f (a, )
(where we used that f does not take the value ) is an open subset of  . Since open sets are
n
measurable, it follows that f is measurable.
Thus, for Lebesgue measure, continuity implies measurability. However, the converse is far
from true because there are many more functions that are measurable than continuous. For
instance, Dirichlet’s function D :   ,

ì 1 if x  ,
D(x) = í
î 0 if x Ï ,
is Lebesgue measurable. Note that D is nowhere continuous. That D is measurable follows from
example below and the fact that D is just the characteristic function of   , and  is measurable.

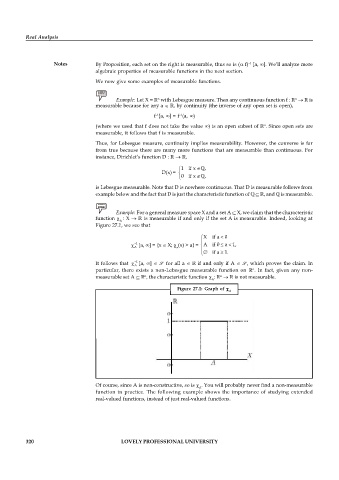
Example: For a general measure space X and a set A  X, we claim that the characteristic
function  : X   is measurable if and only if the set A is measurable. Indeed, looking at
A
Figure 27.1, we see that

ì X if a < 0
ï
-
a
1
 [a, ] = {x  X;  (x) > a} = A if 0 £ < 1,
í
A
A
ï Æ if a ³ 1.
î
1
-
It follows that  [a, ]  S for all a   if and only if A  S , which proves the claim. In
A
n
particular, there exists a non-Lebesgue measurable function on  . In fact, given any non-
measurable set A   , the characteristic function  :    is not measurable.
n
n
A
Figure 27.1: Graph of 
A

Of course, since A is non-constructive, so is  . You will probably never find a non-measurable
A
function in practice. The following example shows the importance of studying extended
real-valued functions, instead of just real-valued functions.

320 LOVELY PROFESSIONAL UNIVERSITY