Page 340 - DMTH401_REAL ANALYSIS

Real Analysis

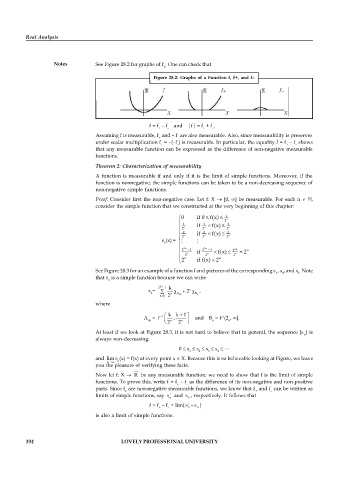
Notes See Figure 28.2 for graphs of f . One can check that
±
Figure 28.2: Graphs of a Function f, f+, and f–

f = f – f and |f| = f + f .
+ – + –
Assuming f is measurable, f and – f are also measurable. Also, since measurability is preserves
+ –
under scalar multiplication f = –(–f ) is measurable. In particular, the equality f = f – f shows
– – + –
that any measurable function can be expressed as the difference of non-negative measurable
functions.
Theorem 2: Characterization of measurability
A function is measurable if and only if it is the limit of simple functions. Moreover, if the
function is nonnegative, the simple functions can be taken to be a non-decreasing sequence of
non-negative simple functions.
Proof: Consider first the non-negative case. Let f: X ® [0, ¥] be measurable. For each n  ,
consider the simple function that we constructed at the very beginning of this chapter:
ì 0 if 0  f(x)  1 n
ï 1 1 2 2
2 ï n if 2 n < f(x)  2 n
ï 2 ï 2 n if 2 2 n < f(x)  2 3 n
s (x) = í  
n
ï
2 ï 2 n - 1 if 2 2 n - 1 < f(x)  2 2 n n
ï 2 n 2 n 2 n = 2
n
ï î 2 n if f(x) > 2 .
See Figure 28.3 for an example of a function f and pictures of the corresponding s , s , and s . Note
1 2 3
that s is a simple function because we can write
n
2 2 n - 1 k
n
s = å c + 2 c ,
n n A B
k 0 2 nk n
=
where
1 k k + ù
- æ 1
–1
A = f , and B = f (2 , ¥].
nk ç n n ú n n
è 2 2 û
At least if we look at Figure 28.3, it is not hard to believe that in general, the sequence {s } is
n
always non-decreasing:
0  s  s  s  s  
1 2 3 4
and lim s (x) = f(x) at every point x  X. Because this is so believable looking at Figure, we leave
n®¥ n
you the pleasure of verifying these facts.
Now let f: X ®  be any measurable function; we need to show that f is the limit of simple
functions. To prove this, write f = f – f as the difference of its non-negative and non-positive
+ –
parts. Since f are non-negative measurable functions, we know that f and f can be written as
± + –
-
+
limits of simple functions, say s and s , respectively. It follows that
n n
-
+
f = f – f = lim( s – s )
+ – n n
is also a limit of simple functions.
334 LOVELY PROFESSIONAL UNIVERSITY