Page 40 - DMTH401_REAL ANALYSIS

Real Analysis

Notes Then again f is a one-one correspondence between S and T.
Such sets are known as equivalent sets. We define the equivalent sets in the following way:
Definition 8: Equivalent Sets
Any two sets are equivalent if there is one-one correspondence between them.

Thus if two sets S and T are equivalent, we write, as S – T.
You can easily show; that S, T and P are any three sets such that S ~ T and T – P, then S – P.
The notion of the equivalent sets is very important because it forms the basis of the ’counting’ of
the infinite sets.
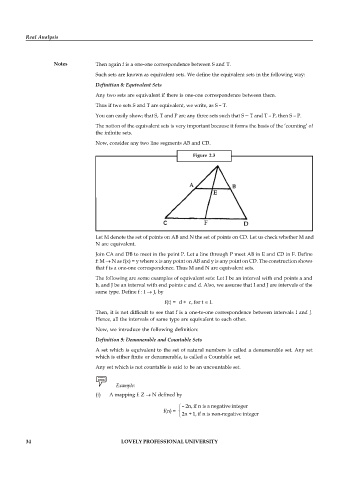
Now, consider any two line segments AB and CD.

Figure 2.3

Let M denote the set of points on AB and N the set of points on CD. Let us check whether M and
N are equivalent.
Join CA and DB to meet in the point P. Let a line through P meet AB in E and CD in F. Define
f: M N as f(x) = y where x is any point on AB and y is any point on CD. The construction shows
that f is a one-one correspondence. Thus M and N are equivalent sets.
The following are some examples of equivalent sets: Let I be an interval with end points a and
b, and J be an interval with end points c and d. Also, we assume that I and J are intervals of the
same type. Define f : I  J, by
f(t) = d + c, for t  I.
Then, it is not difficult to see that f is a one-to-one correspondence between intervals I and J.
Hence, all the intervals of same type are equivalent to each other.
Now, we introduce the following definition:
Definition 9: Denumerable and Countable Sets
A set which is equivalent to the set of natural numbers is called a denumerable set. Any set
which is either finite or denumerable, is called a Countable set.
Any set which is not countable is said to be an uncountable set.

Example:
(i) A mapping f: Z  N defined by

ì – 2n, if n is a negative integer
f(n) = í
î 2n + 1, if n is non-negative integer

34 LOVELY PROFESSIONAL UNIVERSITY