Page 43 - DMTH401_REAL ANALYSIS

Unit 2: Algebraic Structure and Countability

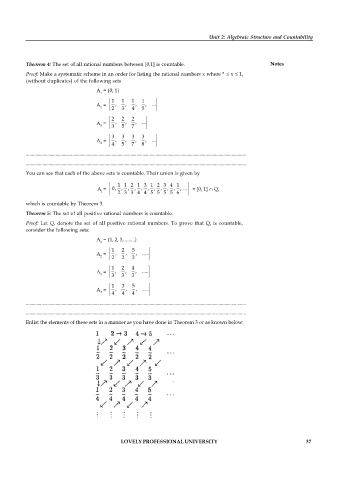
Theorem 4: The set of all rational numbers between [0,1] is countable. Notes
Proof: Make a systematic scheme in an order for listing the rational numbers x where *  x  1,
(without duplicates) of the following sets
A = {0, 1}
1
2 { 1 1 1 1 ,  }
A = 2 , 3 , 4 , 5
A = { 2 , 2 , 2 ,  }
3 3 5 7
4 { 3 , 3 , 3 , 3 ,  }
A = 4 5 7 8

.........................................................................................................................................................................................
.........................................................................................................................................................................................

You can see that each of the above sets is countable. Their union is given by
i { 1 1 2 1 3 1 2 3 4 1 }
,
,
,
,
,
,
,
,
,
A = 0, 2 3 3 4 4 5 5 5 5 6 , = [0, 1]  Q,
which is countable by Theorem 3.
Theorem 5: The set of all positive rational numbers is countable.
Proof: Let Q, denote the set of all positive rational numbers. To prove that Q, is countable,
consider the following sets:
A = {1, 2, 3, ........}
1
2 { 1 2 5 ,  }
A = 2 , 2 , 2 ..
3 { 1 2 4 ,  }
A = 3 , 3 , 3 ..
4 { 1 3 5 ,  }
A = 4 , 4 , 4 ..

.........................................................................................................................................................................................
.........................................................................................................................................................................................
Enlist the elements of these sets in a manner as you have done in Theorem 3 or as known below:

LOVELY PROFESSIONAL UNIVERSITY 37