Page 50 - DMTH401_REAL ANALYSIS

Real Analysis

Notes The word 'topology' is a combination of the two Greek words 'topos' and 'logos'. The term
'topos' means the top or the surface of an object and 'logos' means the study. Thus 'topology'
means the study of surfaces. Since the surfaces are directly related to geometrical objects, therefore
there is a close link between Geometry and Topology. In Geometry, we deal with shapes like
lines, circles, spheres, cubes, cuboids, etc. and their geometrical properties like lengths, areas,
volumes, congruences etc. In Topology, we study the surfaces of these geometrical objects and
certain related properties which are called topological properties. What are these topological
properties of the surfaces of a geometrical figure? We shall not answer this question at this stage.
However, since our discussion is confined to the real line, therefore, we shall discuss this question
pertaining to the topological properties of the real line. These properties are related to the
points and subsets' of the real line such as neighbourhood of a point, open sets, closed sets, limit
points of a set of the real line, etc. We shall, therefore, discuss these notions and concepts in this
unit. However, prior to all these, we discuss the modulus of a real number and its relationship
with the order relations or inequalities.

3.1 Matric Spaces

Definition
A metric space is an ordered pair (M, d) where M is a set and d is a metric on M, i.e., a function
d : M  M 
such that for any x, y, z M, the following holds:
1. d(x, y)  0 (non-negative),

2. d(x, y) = 0 iff x = y (identity of indiscernibles),
3. d(x, y) = d(y, x) (symmetry) and
4. d(x, z)  d(x, y) + d(y, z) (triangle inequality).

The first condition follows from the other three, since:
2d(x, y) = d(x, y) – d(y, x)  d(x, x) = 0
The function d is also called distance function or simply distance. Often, d is omitted and one just
writes M for a metric space if it is clear from the context what metric is used.

Example:

1. The prototype: the line R with its usual distance d(x, y) = |x – y|.
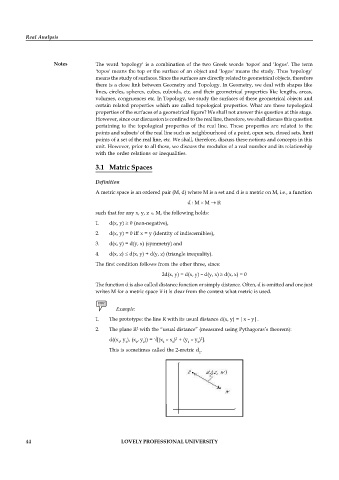
2. The plane R with the “usual distance” (measured using Pythagoras’s theorem):
2
2
2
d((x , y ), (x , y )) = [(x – x ) + (y – y ) ].
1 1 2 2 1 2 1 2
This is sometimes called the 2-metric d .
2

44 LOVELY PROFESSIONAL UNIVERSITY