Page 51 - DMTH401_REAL ANALYSIS

Unit 3: Matric Spaces

3. The same picture will give metric on the complex numbers C interpreted as the Argand Notes
diagram. In this case the formula for the metric is now: d(z, w) = |z – w| where the || in
the formula represent the modulus of the complex number rather than the absolute value
of a real number.
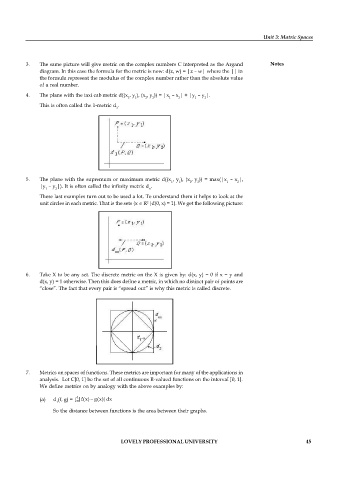
4. The plane with the taxi cab metric d((x , y ), (x , y )) = |x – x | + |y – y |.
1 1 2 2 1 2 1 2
This is often called the 1-metric d .
1

5. The plane with the supremum or maximum metric d((x , y ), (x , y )) = max(|x – x |,
1 1 2 2 1 2
|y – y |). It is often called the infinity metric d .
1 2 
These last examples turn out to be used a lot. To understand them it helps to look at the
2
unit circles in each metric. That is the sets {x R |d(0, x) = 1}. We get the following picture:

6. Take X to be any set. The discrete metric on the X is given by: d(x, y) = 0 if x = y and
d(x, y) = 1 otherwise. Then this does define a metric, in which no distinct pair of points are
“close”. The fact that every pair is “spread out” is why this metric is called discrete.

7. Metrics on spaces of functions. These metrics are important for many of the applications in
analysis. Let C[0, 1] be the set of all continuous R-valued functions on the interval [0, 1].
We define metrics on by analogy with the above examples by:

1
-
(a) d (f, g) = D ò |f(x) g(x)|dx
1
So the distance between functions is the area between their graphs.

LOVELY PROFESSIONAL UNIVERSITY 45