Page 68 - DMTH401_REAL ANALYSIS

Real Analysis

Notes 1
For example, S of S = { : nN} is given by.
n
1 1 1
S = { , nN}{0} = {0, 1, , , .......}
n 2 3
Similarly, you can verify that

Q = Q  Q = Q R = R

R = R  R = R R = R

3.7 Compact Sets

We discuss yet another concept of the so called compactness of a set. The concept of compactness
is formulated in terms of the notion of an open cover of a set.
Definition: Open Cover of a Set
Let S be a set and {G } be a collection of some open subsets of R such that S  G . Then {G} is
 
called an open cover of S.

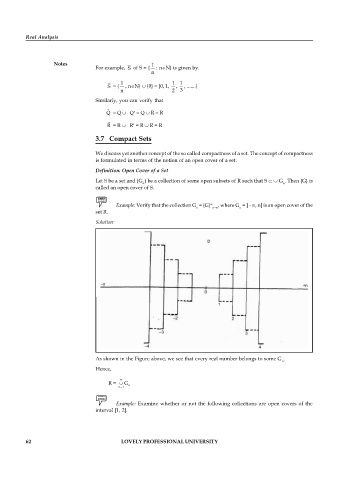
Example: Verify that the collection G = {G}  , where G = ] – n, n[ is an open cover of the
n n= n
set R.
Solution:

As shown in the Figure above, we see that every real number belongs to some G .
n
Hence,


R =  G n
n 1
=
Example: Examine whether or not the following collections are open covers of the
interval [1, 2].

62 LOVELY PROFESSIONAL UNIVERSITY