Page 69 - DMTH401_REAL ANALYSIS

Unit 3: Matric Spaces

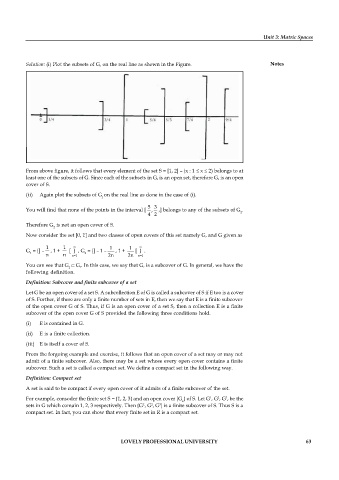
Solution: (i) Plot the subsets of G, on the real line as shown in the Figure. Notes

From above figure, it follows that every element of the set S = [1, 2] – {x : 1  x  2) belongs to at
least one of the subsets of G. Since each of the subsets in G, is an open set, therefore G, is an open
cover of S.
(ii) Again plot the subsets of G on the real line as done in the case of (i).
j
5 3
You will find that none of the points in the interval [ , ,] belongs to any of the subsets of G .
2
4 2
Therefore G is not an open cover of S.
2
Now consider the set [0, 1] and two classes of open covers of this set namely G, and G given as

1 1  1 1 
G = {] – , 1 + [ } , G = {] – 1 – , 1 + [ } .
1 2
n n n 1 2n 2n n 1
=
=
You can see that G G,. In this case, we say that G, is a subcover of G. In general, we have the
2
following definition.
Definition: Subcover and finite subcover of a set
Let G be an open cover of a set S. A subcollection E of G is called a subcover of S if E too is a cover
of S. Further, if there are only a finite number of sets in E, then we say that E is a finite subcover
of the open cover G of S. Thus, if G is an open cover of a set S, then a collection E is a finite
subcover of the open cover G of S provided the following three conditions hold.
(i) E is contained in G.

(ii) E is a finite collection.
(iii) E is itself a cover of S.
From the forgoing example and exercise, it follows that an open cover of a set may or may not
admit of a finite subcover. Also, there may be a set whose every open cover contains a finite
subcover. Such a set is called a compact set. We define a compact set in the following way.
Definition: Compact set

A set is said to be compact if every open cover of it admits of a finite subcover of the set.
2
1
For example, consoder the finite set S = {1, 2, 3} and an open cover {G } of S. Let G , G , G , be the
3

sets in G which contain 1, 2, 3 respectively. Then {G , G , G } is a finite subcover of S. Thus S is a
1
3
2
compact set. In fact, you can show that every finite set in R is a compact set.
LOVELY PROFESSIONAL UNIVERSITY 63