Page 159 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

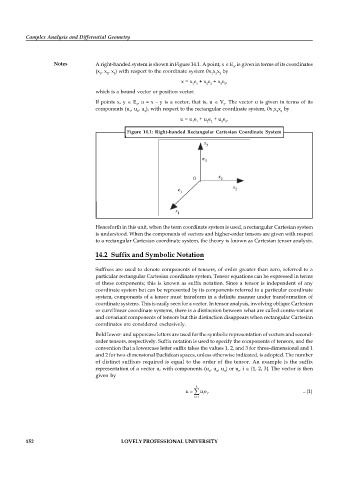
Notes A right-handed system is shown in Figure 14.1. A point, x  E , is given in terms of its coordinates
3
(x , x , x ) with respect to the coordinate system 0x x x by
1 2 3 1 2 3
x = x e + x e + x e ,
3 3
2 2
1 1
which is a bound vector or position vector.
If points x, y  E , u = x – y is a vector, that is, u  V . The vector u is given in terms of its
3
3
components (u , u , u ), with respect to the rectangular coordinate system, 0x x x by
3
1
1 2 3
2
u = u e + u e + u e .
2 2
3 3
1 1
Figure 14.1: Right-handed Rectangular Cartesian Coordinate System

Henceforth in this unit, when the term coordinate system is used, a rectangular Cartesian system
is understood. When the components of vectors and higher-order tensors are given with respect
to a rectangular Cartesian coordinate system, the theory is known as Cartesian tensor analysis.

14.2 Suffix and Symbolic Notation

Suffixes are used to denote components of tensors, of order greater than zero, referred to a
particular rectangular Cartesian coordinate system. Tensor equations can be expressed in terms
of these components; this is known as suffix notation. Since a tensor is independent of any
coordinate system but can be represented by its components referred to a particular coordinate
system, components of a tensor must transform in a definite manner under transformation of
coordinate systems. This is easily seen for a vector. In tensor analysis, involving oblique Cartesian
or curvilinear coordinate systems, there is a distinction between what are called contra-variant
and covariant components of tensors but this distinction disappears when rectangular Cartesian
coordinates are considered exclusively.
Bold lower- and uppercase letters are used for the symbolic representation of vectors and second-
order tensors, respectively. Suffix notation is used to specify the components of tensors, and the
convention that a lowercase letter suffix takes the values 1, 2, and 3 for three-dimensional and 1
and 2 for two-dimensional Euclidean spaces, unless otherwise indicated, is adopted. The number
of distinct suffixes required is equal to the order of the tensor. An example is the suffix
representation of a vector u, with components (u , u , u ) or u , i  {1, 2, 3}. The vector is then
1
2
i
3
given by
3
u   u e . ...(1)
i i
i 1


152 LOVELY PROFESSIONAL UNIVERSITY