Page 285 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes 23.2.2 Tangency of Sphere and Plane

Let S be the oriented sphere with center m and signed radius r, S : (x – m) – r = 0. The tangent
2
2
planes T of S are exactly those planes, whose signed distance from m equals r. Therefore, they
S
satisfy
T : n m + n m + n m + d = n . m + d = r. (10)
1
S
1
3
3
2
2
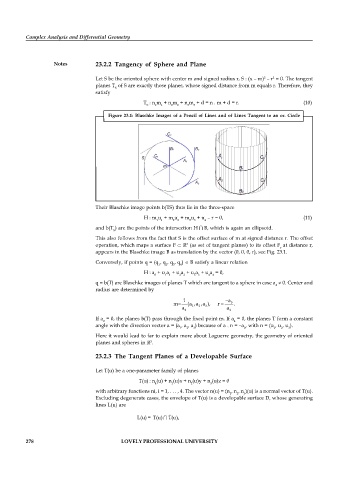
Figure 23.1: Blaschke Images of a Pencil of Lines and of Lines Tangent to an or. Circle

Their Blaschke image points b(TS) thus lie in the three-space

H : m u + m u + m u + u – r = 0, (11)
2
1
2
4
1
3
3
and b(T ) are the points of the intersection H  B, which is again an ellipsoid.
S
This also follows from the fact that S is the offset surface of m at signed distance r. The offset
operation, which maps a surface F   (as set of tangent planes) to its offset F at distance r,
3
r
appears in the Blaschke image B as translation by the vector (0, 0, 0, r), see Fig. 23.1.
Conversely, if points q = (q , q , q , q )  B satisfy a linear relation
1
4
3
2
H : a + u a + u a + u a + u a = 0,
4 4
3 3
2 2
0
1 1
q = b(T) are Blaschke images of planes T which are tangent to a sphere in case a  0. Center and
4
radius are determined by
1  a
m= (a ,a ,a ), r  0 .
a 4 1 2 3 a 4
If a = 0, the planes b(T) pass through the fixed point m. If a = 0, the planes T form a constant
4
0
angle with the direction vector a = (a , a , a ) because of a . n = –a , with n = (u , u , u ).
3
3
0
2
1
2
1
Here it would lead to far to explain more about Laguerre geometry, the geometry of oriented
planes and spheres in  .
3
23.2.3 The Tangent Planes of a Developable Surface
Let T(u) be a one-parameter family of planes
T(u) : n (u) + n (u)x + n (u)y + n (u)z = 0
2
4
3
1
with arbitrary functions ni, i = 1, . . . , 4. The vector n(u) = (n , n , n )(u) is a normal vector of T(u).
3
1
2
Excluding degenerate cases, the envelope of T(u) is a developable surface D, whose generating
lines L(u) are

L(u) = T(u)  T(u),
278 LOVELY PROFESSIONAL UNIVERSITY