Page 317 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes These two approaches to the curvature are related geometrically by the following observation.
In the first definition, the curvature of a circle is equal to the ratio of the angle of an arc to its
length. Likewise, the curvature of a plane curve at any point is the limiting ratio of d, an
infinitesimal angle (in radians) between tangents to that curve at the ends of an infinitesimal
segment of the curve, to the length of that segment ds, i.e., d/ds. If the tangents at the ends of the
segment are represented by unit vectors, it is easy to show that in this limit, the magnitude of the
difference vector is equal to d, which leads to the given expression in the second definition of
curvature.
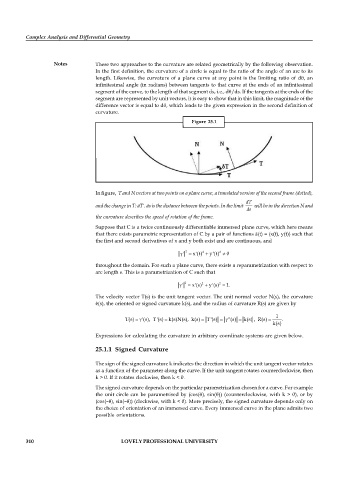
Figure 25.1

In figure, T and N vectors at two points on a plane curve, a translated version of the second frame (dotted),
dT
and the change in T: äT’. äs is the distance between the points. In the limit will be in the direction N and
ds
the curvature describes the speed of rotation of the frame.

Suppose that C is a twice continuously differentiable immersed plane curve, which here means
that there exists parametric representation of C by a pair of functions ã(t) = (x(t), y(t)) such that
the first and second derivatives of x and y both exist and are continuous, and

2
2
'  2  x'(t)  y'(t)  0
throughout the domain. For such a plane curve, there exists a reparametrization with respect to
arc length s. This is a parametrization of C such that

2
2
'  2  x'(s)  y'(s)  1.
The velocity vector T(s) is the unit tangent vector. The unit normal vector N(s), the curvature
ê(s), the oriented or signed curvature k(s), and the radius of curvature R(s) are given by
1
T(s)   '(s), T'(s) k(s)N(s), k(s)  T'(s)   ''(s)  k(s) , R(s)  .

k(s)
Expressions for calculating the curvature in arbitrary coordinate systems are given below.

25.1.1 Signed Curvature

The sign of the signed curvature k indicates the direction in which the unit tangent vector rotates
as a function of the parameter along the curve. If the unit tangent rotates counterclockwise, then
k > 0. If it rotates clockwise, then k < 0.
The signed curvature depends on the particular parametrization chosen for a curve. For example
the unit circle can be parametrised by (cos(), sin()) (counterclockwise, with k > 0), or by
(cos(–), sin(–)) (clockwise, with k < 0). More precisely, the signed curvature depends only on
the choice of orientation of an immersed curve. Every immersed curve in the plane admits two
possible orientations.

310 LOVELY PROFESSIONAL UNIVERSITY