Page 320 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 25: Curvature

Here the t denotes the matrix transpose. This last formula is also valid for the curvature of curves Notes
in a Euclidean space of any dimension.

25.2.2 Curvature from Arc and Chord Length

Given two points P and Q on C, let s(P,Q) be the arc length of the portion of the curve between
P and Q and let d(P,Q) denote the length of the line segment from P to Q. The curvature of C at
P is given by the limit
24(s(P,Q) d(P,Q))

k(P) lim

Q P s(P,Q) 3
where the limit is taken as the point Q approaches P on C. The denominator can equally well be
taken to be d(P,Q) . The formula is valid in any dimension. Furthermore, by considering the
3
limit independently on either side of P, this definition of the curvature can sometimes
accommodate a singularity at P. The formula follows by verifying it for the osculating circle.

25.3 Curves on Surfaces

When a one dimensional curve lies on a two dimensional surface embedded in three dimensions
R , further measures of curvature are available, which take the surface’s unit-normal vector, u
3
into account. These are the normal curvature, geodesic curvature and geodesic torsion. Any non-
singular curve on a smooth surface will have its tangent vector T lying in the tangent plane of
the surface orthogonal to the normal vector. The normal curvature, k , is the curvature of the
n
curve projected onto the plane containing the curve’s tangent T and the surface normal u; the
geodesic curvature, k , is the curvature of the curve projected onto the surface’s tangent plane;
g
and the geodesic torsion (or relative torsion), ô , measures the rate of change of the surface
r
normal around the curve’s tangent.
Let the curve be a unit speed curve and let t = u × T so that T, u, t form an orthonormal basis: the
Darboux frame. The above quantities are related by:

 T'   o K K   T 
   g n   
t
 t'   K g o T n  

 
 u'    K  T o   
u
   n   
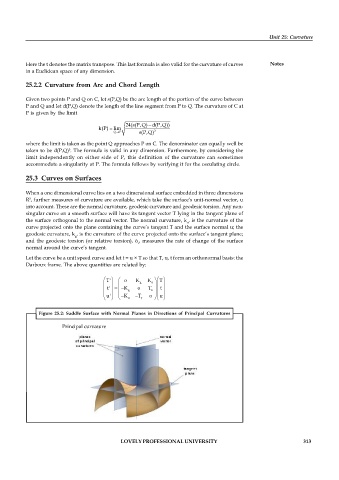
Figure 25.2: Saddle Surface with Normal Planes in Directions of Principal Curvatures
Principal curvature

LOVELY PROFESSIONAL UNIVERSITY 313