Page 389 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

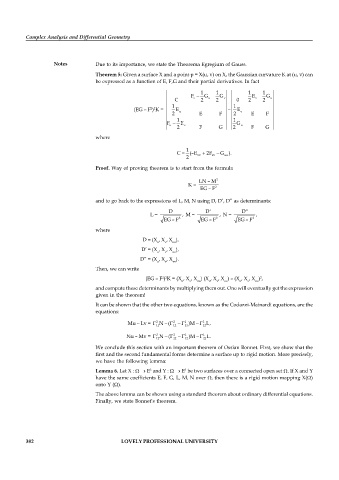
Notes Due to its importance, we state the Theorema Egregium of Gauss.
Theorem 5: Given a surface X and a point p = X(u, v) on X, the Gaussian curvature K at (u, v) can
be expressed as a function of E, F,G and their partial derivatives. In fact
1 G 1 G 1 E 1 G
v
C F  2 u 2 v 0 2 v 2 u
1 1
(EG – F ) K = E  E
2 2
2 u E F 2 v E F
1 1
F  2 E v F G 2 G u F G
u
where
1
C  (–E vv  2F  G ).
uu
vv
2
Proof. Way of proving theorem is to start from the formula
LN – M 2
K =
EG – F 2
and to go back to the expressions of L, M, N using D, D’, D” as determinants:

L = D , M = D' , N = D" ,
EG – F 2 EG – F 2 EG – F 2
where

D = (X , X , X ),
uu
v
u
D’ = (X , X , X ),
v
uv
u
D” = (X , X , X ).
v
u
vv
Then, we can write
(EG – F ) K = (X , X , X ) (X , X , X ) – (X , X , X ) ,
2
2 2
u
uu
v
v
u
uv
v
vv
u
and compute these determinants by multiplying them out. One will eventually get the expression
given in the theorem!
It can be shown that the other two equations, known as the Codazzi-Mainardi equations, are the
equations:
2

Mu – Lv =  2 11 N (   1 11 )M   1 12 L,
12
2

Nu – Mv =  2 12 N (   1 12 )M   1 22 L.
22
We conclude this section with an important theorem of Ossian Bonnet. First, we show that the
first and the second fundamental forms determine a surface up to rigid motion. More precisely,
we have the following lemma:
Lemma 6. Let X :   E and Y :   E be two surfaces over a connected open set . If X and Y
3
3
have the same coefficients E, F, G, L, M, N over , then there is a rigid motion mapping X()
onto Y ().
The above lemma can be shown using a standard theorem about ordinary differential equations.
Finally, we state Bonnet’s theorem.
382 LOVELY PROFESSIONAL UNIVERSITY