Page 49 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes Emotionally, the fact that two closed curves are homotopic in D means that one can be
continuously deformed into the other in D.

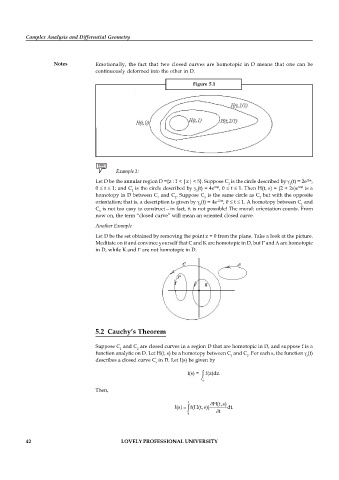
Figure 5.1

Example 1:
Let D be the annular region D ={z : 1 < |z| < 5}. Suppose C is the circle described by  (t) = 2e ,
i2t
1
1
0  t  1; and C is the circle described by  (t) = 4e , 0  t  1. Then H(t, s) = (2 + 2s)e is a
i2t
i2t
2
2
homotopy in D between C and C . Suppose C is the same circle as C but with the opposite
2
2
3
1
orientation; that is, a description is given by  (t) = 4e –i2t , 0  t  1. A homotopy between C and
1
3
C is not too easy to construct—in fact, it is not possible! The moral: orientation counts. From
3
now on, the term “closed curve” will mean an oriented closed curve.
Another Example
Let D be the set obtained by removing the point z = 0 from the plane. Take a look at the picture.
Meditate on it and convince yourself that C and K are homotopic in D, but  and  are homotopic
in D, while K and  are not homotopic in D.

5.2 Cauchy’s Theorem

Suppose C and C are closed curves in a region D that are homotopic in D, and suppose f is a
1
2
function analytic on D. Let H(t, s) be a homotopy between C and C . For each s, the function  (t)
2
1
s
describes a closed curve C in D. Let I(s) be given by
s
I(s) =  f(z)dz.
C s
Then,
1  H(t,s)
I(s)   f(H(t,s)) dt.
0 t 

42 LOVELY PROFESSIONAL UNIVERSITY