Page 106 - DMTH403_ABSTRACT_ALGEBRA

Unit 8: Permutation Groups

Thus, by the Fundamental Theorem of Homomorphism, Notes
G/Ker f  Im f  S(G),

that is, G is isomorphic to a subgroup of S(G).
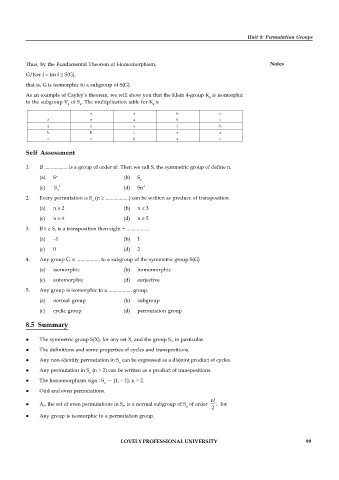
As an example of Cayley’s theorem, we will show you that the Klein 4-group K is isomorphic
4
to the subgroup V of S . The multiplication table for K is
4
4
4
. e a b c
e e a b c
a a e c b
b b c e a
c c b a e
Self Assessment

1. If .................. is a group of order n!. Then we call S, the symmetric group of define n.
(a) S n (b) S n
(c) S  n 1 (d) Sn -1
2. Every permutation is S (n  ..................) can be written as produce of transposition
n
(a) n  2 (b) n  3
(c) n  4 (d) n  5
3. If t  S, is a transposition then sight = ..................
(a) –1 (b) 1

(c) 0 (d) 2
4. Any group G is .................. to a subgroup of the symmetric group S(G)
(a) isomorphic (b) homomorphic
(c) automorphic (d) surjective

5. Any group is isomorphic to a .................. group.
(a) normal group (b) subgroup
(c) cyclic group (d) permutation group

8.5 Summary

The symmetric group S(X), for any set X, and the group S,, in particular.

The definitions and some properties of cycles and transpositions.

Any non-identity permutation in S can be expressed as a disjoint product of cycles.
 n
Any permutation in S (n  2) can be written as a product of transpositions.
 n
The homomorphism sign : S — {1, – 1}, n  2.
 n
Odd and even permutations.

n!
A,, the set of even permutations in S,, is a normal subgroup of S of order , for
 n 2
Any group is isomorphic to a permutation group.


LOVELY PROFESSIONAL UNIVERSITY 99