Page 48 - DMTH403_ABSTRACT_ALGEBRA

Unit 3: Subgroups

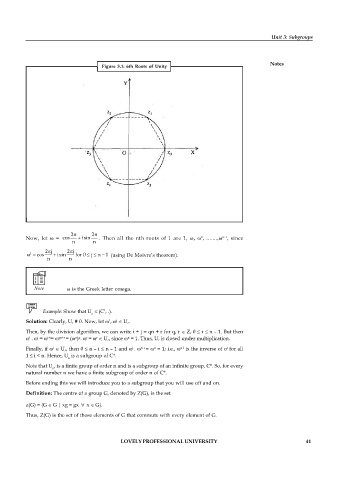
Figure 3.1: 6th Roots of Unity Notes

Now, let  = cos 2  isin 2 . Then all the nth roots of 1 are 1, ,  , ......., , since
n-1
2
n n
2 j 2 j


j
j
  cos  isin for 0   n 1 (using De Moivre’s theorem).

n n
Note  is the Greek letter omega.

Example: Show that U  (C’, .).
n
Solution: Clearly, U, # 0. Now, let  ,   U,.
1
j
Then, by the division algorithm, we can write i + j = qn + r for q, r  Z, 0  r  n – 1. But then
i
r
n q
r
n
i+j
j
 .  =  =  qn+r = ( ) .  =   U,, since  = 1. Thus, U, is closed under multiplication.
Finally, if   U,, then 0  n – i  n – 1 and  .  =  = 1; i.e.,  is the inverse of o for all
n–i
i
i
n-i
i
n
1  i < n. Hence, U is a subgroup of C*.
n
Note that U , is a finite group of order n and is a subgroup of an infinite group, C*. So, for every
n
natural number n we have a finite subgroup of order n of C*.
Before ending this we will introduce you to a subgroup that you will use off and on.
Definition: The centre of a group G, denoted by Z(G), is the set
z(G) = {G  G | xg = gx  x  G}.
Thus, Z(G) is the set of those elements of G that commute with every element of G.
LOVELY PROFESSIONAL UNIVERSITY 41