Page 257 - DMTH404_STATISTICS

Unit 17: System Reliability

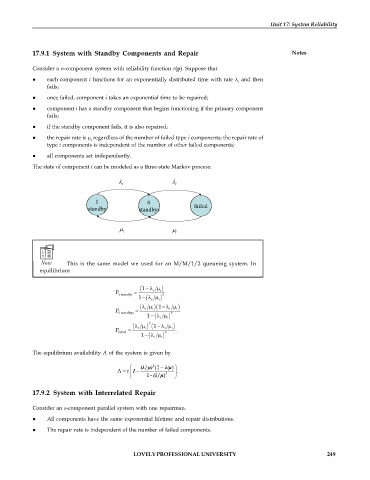
17.9.1 System with Standby Components and Repair Notes

Consider a n-component system with reliability function r(p). Suppose that
 each component i functions for an exponentially distributed time with rate  and then
i
fails;
 once failed, component i takes an exponential time to be repaired;
 component i has a standby component that begins functioning if the primary component
fails;

 if the standby component fails, it is also repaired;
 the repair rate is  regardless of the number of failed type i components; the repair rate of
i
type i components is independent of the number of other failed components;
 all components act independently.
The state of component i can be modeled as a three-state Markov process:

 i  i

1 0
standby standbys failed

 i  i

Note This is the same model we used for an M/M/1/2 queueing system. In
equilibrium

1    
P  i i
1 standby 3
1    i 
i
  i 1    i 
i
i
P 
0 standbys 3
1    i 
i
2
   1    
P  i i i i .
failed 3
1    i 
i
The equilibrium availability A of the system is given by
 (λ /μ) 2 (1  λ/μ ) 
r
A   1  3 . 
μ
 1 - (λ / ) 
17.9.2 System with Interrelated Repair
Consider an s-component parallel system with one repairman.
 All components have the same exponential lifetime and repair distributions.
 The repair rate is independent of the number of failed components.

LOVELY PROFESSIONAL UNIVERSITY 249