Page 136 - DMTH502_LINEAR_ALGEBRA

Linear Algebra

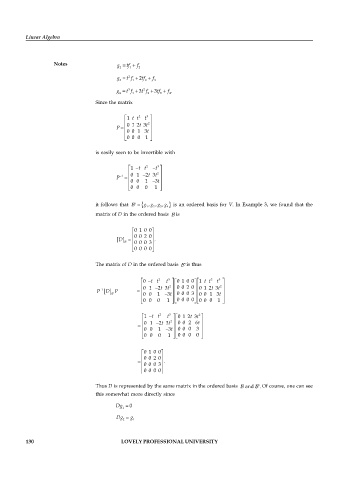
Notes g tf f
2 1 2
2
g 3 t f 1 2tf 2 f 3
3
2
g 4 t f 1 3t f 2 3tf 3 4 . f
Since the matrix

1 t t 2 t 3
0 1 2 3t 2
t
P
0 0 1 3t
0 0 0 1
is easily seen to be invertible with

1 t t 2 t 3
t
0 1 2 3t 2
P 1
0 0 1 3t
0 0 0 1
it follows that  ' g 1 , g g g is an ordered basis for V. In Example 3, we found that the
,
,
4
3
2
matrix of D in the ordered basis  is
0 1 0 0
0 0 2 0
D .
 0 0 0 3
0 0 0 0

The matrix of D in the ordered basis ' is thus

0 t t 2 t 3 0 1 0 0 1 t t 2 t 3
t
0 1 2 3t 2 0 0 2 0 0 1 2 3t t 2
P 1 D P 0 0 1 3t 0 0 0 3 0 0 1 3t

0 0 0 1 0 0 0 0 0 0 0 1
t
1 t t 2 t 3 0 1 2 3t 2
t
0 1 2 3t 2 0 0 2 6t
0 0 1 3t 0 0 0 3
0 0 0 1 0 0 0 0

0 1 0 0
0 0 2 0
0 0 0 3 .
0 0 0 0


Thus D is represented by the same matrix in the ordered basis  and '. Of course, one can see
this somewhat more directly since
Dg 0
1
Dg 2 g 1

130 LOVELY PROFESSIONAL UNIVERSITY