Page 330 - DMTH502_LINEAR_ALGEBRA
P. 330
Linear Algebra
Notes 29.5 Review Questions
1. If T is a normal operator, prove that characteristic vectors for T which are associated with
distinct characteristic values are orthogonal.
2. Let T be a linear operator on the finite dimensional complex inner product space V. Prove
that the following statements about T are equivalent.
(a) T is normal
(b) T = T* for every in V
(c) If is a vector and c a scalar such that T = c , then T* = c .
(d) There is an orthonormal basis such that [T] is diagonal.
Answer: Self Assessment
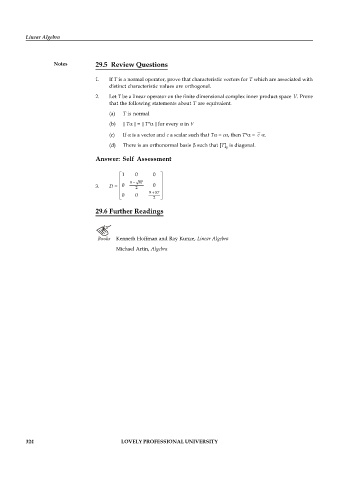
1 0 0
9 57
3. D = 0 2 0
9 57
0 0
2
29.6 Further Readings
Books Kenneth Hoffman and Ray Kunze, Linear Algebra
Michael Artin, Algebra
324 LOVELY PROFESSIONAL UNIVERSITY