Page 261 - DMTH503_TOPOLOGY

Unit 32: Introduction to Dimension Theory

Notes

x

r

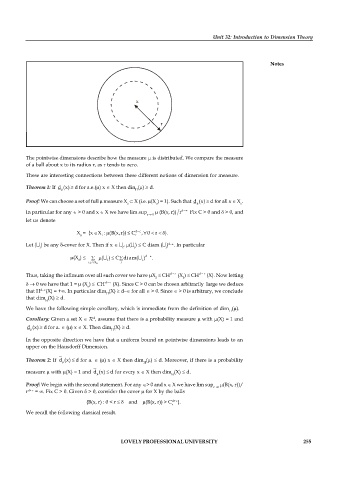
The pointwise dimensions describe how the measure  is distributed. We compare the measure
of a ball about x to its radius r, as r tends to zero.
These are interesting connections between these different notions of dimension for measure.

Theorem 1: If d (x) d³ for a.e.() xX then din () ³ d.
 H
Proof: We can choose a set of full  measure X X (i.e.(X ) = 1). Such that d (x) d³ for all xX .
o o  o
In particular for any > 0 and xX we have lim sup (B(x, r)) r d- Fix C > 0 and  > 0, and
r0
let us denote

X = {x X : (B(x,r)) C   d- , 0 < <  }.
r

 i r
Let { } be any -cover for X. Then if x  , ( )  C diam ( ) d– . In particular
i i i i
(X )  å (  ) C diam( ) d -  .
 å
 i i
 i Ç X  i
Thus, taking the infimum over all such cover we have X  CH d - (X )  CH d - (X). Now letting
 
  0 we have that 1 =  (X )  CH d - (X). Since C > 0 can be chosen arbitrarily large we deduce
o
that H d– (X) = +¥. In particular dim (X) ³ d– for all> 0. Since> 0 is arbitrary, we conclude
H
that dim (X)³d.
H
We have the following simple corollary, which is immediate from the definition of dim ().
H
Corollary: Given a set X , assume that there is a probability measurewith(X) = 1 and
d
d (x) d for a.() xX. Then dim (X)³d.
³

H
In the opposite direction we have that a uniform bound on pointwise dimensions leads to an
upper on the Hausdorff Dimension.
Theorem 2: If d (x) d for a.() xX then dim ()d. Moreover, if there is a probability
 H
measurewith(X) = 1 and d (x) d for every xX then dim (X)d.
 H
Proof: We begin with the second statement. For any > 0 and xX we have lim sup (B(x, r))/
r0
r d+ =¥. Fix C > 0. Given  > 0, consider the coverfor X by the balls
{B(x, r) : 0 < r and (B(x, r)) > C d+ }.
r
We recall the following classical result.

LOVELY PROFESSIONAL UNIVERSITY 255