Page 11 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes 2
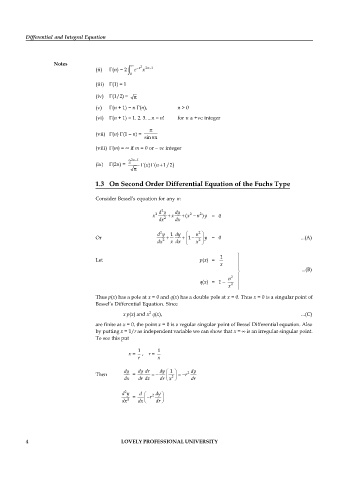
(ii) (n) = 2 e x x 2n 1
0
(iii) (1) = 1

(iv) (1/2) =
(v) (n + 1) = n (n), n > 0
(vi) (n + 1) = 1. 2. 3. ...n = n! for n a +ve integer

(vii) (n) (1 n) =
sinn
(viii) (m) = if m = 0 or ve integer

2 2n 1
(ix) (2n) = ( ) n 1/2
x

1.3 On Second Order Differential Equation of the Fuchs Type
,
Consider Bessel s equation for any n:

2
2 d y dy 2 2
x x (x n )y = 0
dx 2 dx
2
d y 1 dy n 2
Or 2 1 2 y = 0 ...(A)
dx x dx x
1
Let p(x) =
x
...(B)
n 2
q(x) = 1
x 2
Thus p(x) has a pole at x = 0 and q(x) has a double pole at x = 0. Thus x = 0 is a singular point of
,
Bessel s Differential Equation. Since
2
x p(x) and x q(x), ...(C)
are finite at x = 0, the point x = 0 is a regular singular point of Bessel Differential equation. Also
by putting x = 1/r as independent variable we can show that x = is an irregular singular point.
To see this put
1 1
x = , r =
r x

dy dy dr dy 1 2 dy
Then = 2 r
dx dr dx dr x dr

2
d y = d r 2 dy
dx 2 dx dr

4 LOVELY PROFESSIONAL UNIVERSITY