Page 297 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

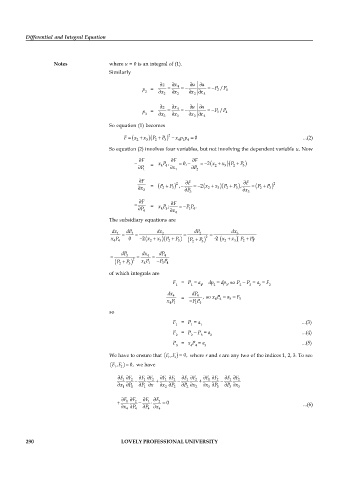
Notes where u = 0 is an integral of (1).
Similarly

z x 4 u u
p = P 2 /P 4
2 x 2 x 2 x 2 x 4

z x 4 u u
p = P 3 /P 4
3 x 3 x 3 x 3 x 4
So equation (1) becomes
2
F x x P P x p p 0 ...(2)
2 3 2 3 4 1 4
So equation (2) involves four variables, but not involving the dependent variable u. Now

F F F
= x P , 0, 2 x 2 x 3 P 2 P 3
4 4
P x P
1 1 2
F 2 F F 2
= P P , 2 x x P P , P P
x 2 2 3 P 3 2 3 2 3 x 3 2 3
F F
P 4 = x P ; x 4 P P .
4 1
1 4
The subsidiary equations are
dx 1 dP 1 dx 2 dP 2 dx 3
x P 0 2 x x P P 2 2 x x (P P )
4 4 2 3 2 3 P 2 P 3 2 3 2 3
dP 3 dx 4 dP 4
2
P 2 P 3 x P P P
1 4
4 1
of which integrals are
F = P = a , dp = dp , so P  P = a = F
1 1 1 2 3 2 3 2 2
dx 4 dP 4
4 4
x P = P P , so x P a 3 F 3
4 1 1 4
so
F = P = a ...(3)
1 1 1
F = P  P = a ...(4)
2 2 3 2
P = x P = a ...(5)
3 4 4 3
We have to ensure that F r ,F s 0, where r and s are any two of the indices 1, 2, 3. To see

F ,F 0, we have
1 2
F 1 F 2 F 1 F 2 F 1 F 2 F 1 F 2 F 1 F 2 F 1 F 2
x 1 P 1 P 1 x x 2 P 2 P 2 x 2 x 3 P 3 P 3 x 3

F F F F
1 2 1 2 0
x 4 P 4 P 4 x 4 ...(6)

290 LOVELY PROFESSIONAL UNIVERSITY