Page 299 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

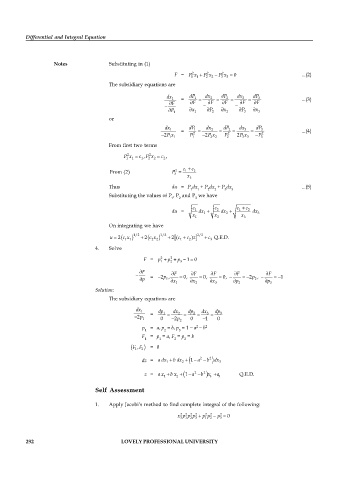
Notes Substituting in (1)
2
2
2
F = P x 1 P x 2 P x 3 0 ...(2)
1
2
3
The subsidiary equations are
dx 1 = dP 1 dx 2 dP 2 dx 3 dP 3 ...(3)
F F F F F F
P 1 x 1 P 2 x 2 P 3 x 3
or
dx 1 dP 1 dx 2 dP 2 dx 3 dP 3
2P x = P 2 2P x P 2 2P x P 2 ...(4)
1 1 1 2 2 2 3 3 3
From first two terms

2
2
P x 1 c 1 ,P x 2 c 2 ,
2
1
c c
From (2) P 2 1 2
3
x
3
Thus du = P dx + P dx + P dx ...(5)
1 1 2 2 3 3
Substituting the values of P , P and P we have
1 2 3
c c c c
du = 1 dx 1 2 dx 2 1 2 dx 3
x 1 x 2 x 3
On integrating we have
1/2 1/2 1/2
u 2 c x 2 c x 2 (c 1 c 2 )z c 3 Q.E.D.
2 2
1 1
4. Solve
F = p 2 1 p 2 2 p 3 1 0
F F F F F F
= 2p , 0, 0, 0, 2p , 1
p 1 x x x p 2 p
1 2 3 2 3
Solution:
The subsidiary equations are
dx 1 dp 1 dx 2 dp 2 dx 3 dp 3
2p 1 = 0 2p 2 0 1 0
2
p = a, p = b, p = 1  a  b 2
1 2 3
F = p = a, F = p = b
1 1 2 2
F 1 ,F 2 = 0
2
dz = a dx 1 b dx 2 1 a 2 b dx 3
2
z = a x 1 b x 2 1 a 2 b x 3 a 3 Q.E.D.

Self Assessment

1. Apply Jacobi’s method to find complete integral of the following:
2 2
2 2 2 2
x p p p p p p 3 2 0
3 1 2 3
1 2
292 LOVELY PROFESSIONAL UNIVERSITY