Page 356 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 21: Classifications of Second Order Partial Differential Equations

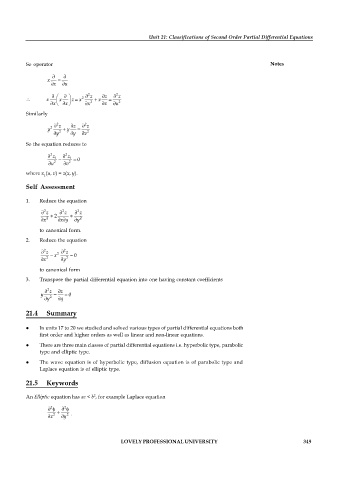
So operator Notes

x
x u
2 z z 2 z
x x z x 2 x
x x x 2 x u 2
Similarly

2 z z 2 z
y 2 y
y 2 y v 2
So the equation reduces to

2 2
z 1 z 1 0
u 2 v 2
where z (u, v) = z(x, y).
1
Self Assessment

1. Reduce the equation
2 2 2
z 2 z z
x 2 x y y 2
to canonical form.
2. Reduce the equation
2 2
z x 2 z 0
x 2 y 2

to canonical form
3. Transpose the partial differential equation into one having constant coefficients
2 z z
y 2 0
y q

21.4 Summary

 In units 17 to 20 we studied and solved various types of partial differential equations both
first order and higher orders as well as linear and non-linear equations.
 There are three main classes of partial differential equations i.e. hyperbolic type, parabolic
type and elliptic type.
 The wave equation is of hyperbolic type, diffusion equation is of parabolic type and
Laplace equation is of elliptic type.

21.5 Keywords

2
An Elliptic equation has ac < b , for example Laplace equation
2 2
 
x 2 y 2 .

LOVELY PROFESSIONAL UNIVERSITY 349