Page 186 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 15: Banach Space: Definition and Some Examples

Notes
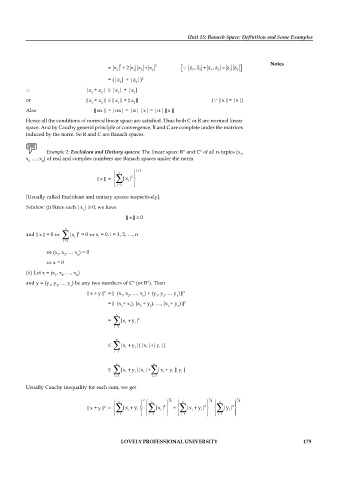
2 2
= z 1 2 z z 2 z 2  z , z 2 z , z 2 z z 2
1
1
1
1
= (|z | + |z |) 2
1 2
|z + z | |z | + |z |
1 2 1 2
or z + z z + z ( x = |x|)
1 2 1 2
Also x = | x| = | | |x| = | | x
Hence all the conditions of normed linear space are satisfied. Thus both C or R are normed linear
space. And by Cauchy general principle of convergence, R and C are complete under the matrices
induced by the norm. So R and C are Banach spaces.

n
n
Example 2: Euclidean and Unitary spaces: The linear space R and C of all n-tuples (x ,
1
x …, x ) of real and complex numbers are Banach spaces under the norm
2 n
1/2
n
x = |x | 2
i
i 1
[Usually called Euclidean and unitary spaces respectively].
Solution: (i) Since each |x | 0, we have
i
x 0

n
2
and x = 0 |x | = 0 x = 0, i = 1, 2, …, n
i i
i 1
(x , x , … x ) = 0
1 2 n
x = 0
(ii) Let x = (x , x , …, x )
1 2 n
n
n
and y = (y , y , … y ) be any two numbers of C (or R ). Then
1 2 n
x + y 2 = (x , x , …, x ) + (y , y , … y ) 2
1 2 n 1 2 n
= (x + x ), (x + y ), …, (x + y ) 2
1 1 2 2 n n
n
= |x y | 2
i i
i 1
n
|x y |(|x | |y |)
i i i i
i 1

n n
|x y ||x | |x y ||y |
i i i i i i
i 1 i 1
Usually Cauchy inequality for each sum, we get

2 1 1 1
n n 2 n 2 n 2
x + y 2 = |x i y | |x | 2 |x i y | 2 |y | 2
i
i
i
i
i 1 i 1 i 1 i 1
LOVELY PROFESSIONAL UNIVERSITY 179