Page 35 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

Notes
x x o
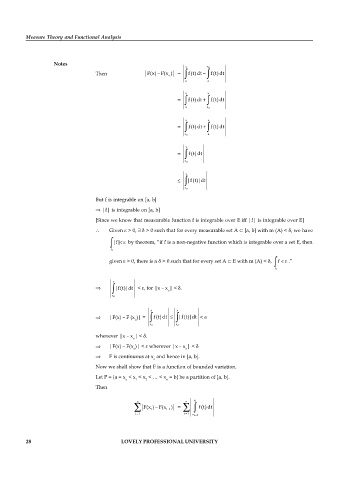
Then F(x) F(x ) = f(t) dt f(t) dt
o
a a
x a
= f(t) dt f(t) dt
a x o

a x
= f(t) dt f(t) dt
x o a

x
= f(t) dt
x o

x
|f(t)|dt
x o
But f is integrable on [a, b]

|f| is integrable on [a, b]
[Since we know that measurable function f is integrable over iff |f| is integrable over E]
Given > 0, > 0 such that for every measurable set A [a, b] with m (A) < , we have

|f| by theorem, “if f is a non-negative function which is integrable over a set E, then
A

given > 0, there is a > 0 such that for every set A E with m (A) < , f .”
A

x
|f(t)|dt < , for |x – x | < .
o
x o

x x
|F(x) – F (x )| = f(t) dt |f(t)|dt <
o
x o x o
whenever |x – x | < .
o
|F(x) – F(x )| < wherever |x – x | <
o o
F is continuous at x and hence in [a, b].
o
Now we shall show that F is a function of bounded variation.
Let P = {a = x < x < x < … < x = b} be a partition of [a, b].
o 1 2 n
Then

n n x i
F(x ) F(x ) = f(t) dt
i 1
i
i 1 i 1 x i 1

28 LOVELY PROFESSIONAL UNIVERSITY