Page 38 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 3: Differentiation of an Integral

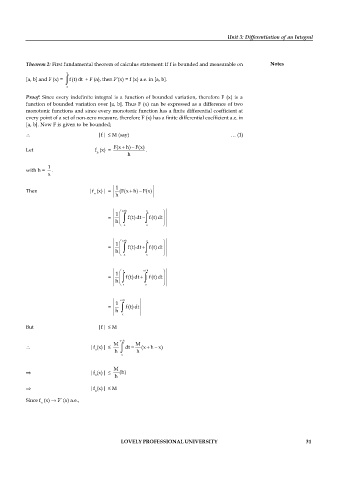
Theorem 2: First fundamental theorem of calculus statement: If f is bounded and measurable on Notes
x
[a, b] and F (x) = f(t) dt + F (a), then F (x) = f (x) a.e. in [a, b].
a
Proof: Since every indefinite integral is a function of bounded variation, therefore F (x) is a
function of bounded variation over [a, b]. Thus F (x) can be expressed as a difference of two
monotonic functions and since every monotonic function has a finite differential coefficient at
every point of a set of non-zero measure, therefore F (x) has a finite differential coefficient a.e. in
[a, b]. Now F is given to be bounded;
|f| M (say) … (1)

F(x h) F(x)
Let f (x) = .
n
h
1
with h = .
x

1
Then |f (x)| = (F(x h) F(x)
n
h
x h x
1
= f(t) dt f(t) dt
h
a a
x h a
1
= f(t) dt f(t) dt
h
a x

a x h
1
= f(t) dt f(t) dt
h
x a

x h
1
= f(t) dt
h
x
But |f| M

x h
M M
|f (x)| dt (x h x)
n
h h
x
M
|f (x)| (h)
n h

|f (x)| M
n
Since f (x) F (x) a.e.,
n

LOVELY PROFESSIONAL UNIVERSITY 31