Page 47 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

Notes
n n
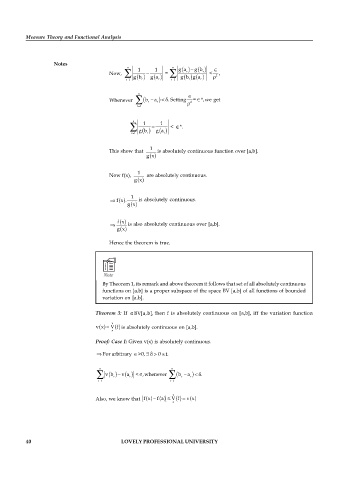
1 1 g a r g b r
Now, = < 2 ,
g b g a g b g a
r 1 r r r 1 r r
n
Whenever b r a r . Setting 2 = *,we get
r 1

n
1 1
< *.
g b g a
r 1 r r
1
This show that is absolutely continuous function over [a,b].
g x

1
Now f(x), are absolutely continuous.
g x

1
f x . is absolutely continuous.
g x

f x
is also absolutely continuous over [a,b].
g x

Hence the theorem is true.

Note
By Theorem 1, its remark and above theorem it follows that set of all absolutely continuous
functions on [a,b] is a proper subspace of the space BV [a,b] of all functions of bounded
variation on [a,b].

Theorem 3: If BV[a,b], then f is absolutely continuous on [a,b], iff the variation function

x
v x V f is absolutely continuous on [a,b].
a
Proof: Case I: Given v(x) is absolutely continuous.

For arbitrary >0, 0 s.t.

n n
v b v a < ,whenever b a .
r r r r
r 1 r 1
x
Also, we know that f x f a V f v x
a

40 LOVELY PROFESSIONAL UNIVERSITY