Page 201 - DSOC404_METHODOLOGY_OF_SOCIAL_RESEARCH_ENGLISH

Unit-27: Measures of Central Tendency: Mean, Median, Mode

note
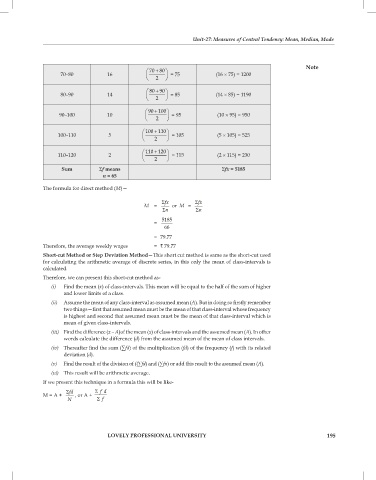
 70 80  +
70–80 16   = 75 (16 × 75) = 1200
 2 
 80 90  +
80–90 14   = 85 (14 × 85) = 1190
 2 
 90 100  +
90–100 10   = 95 (10 × 95) = 950
 2 

 + 110  100
100–110 5   = 105 (5 × 105) = 525
 2 
 + 120  110
110–120 2   = 115 (2 × 115) = 230
 2 
sum Sf means Sfx = 5185
n = 65

the formula for direct method (M)—

S fx S fx
M = or M =
S n S n
5185
=
66
= 79.77
therefore, the average weekly wages = ` 79.77
Short-cut Method or Step Deviation Method—this short cut method is same as the short-cut used
for calculating the arithmetic average of discrete series, in this only the mean of class-intervals is
calculated.
therefore, we can present this short-cut method as-
(i) find the mean (x) of class-intervals. this mean will be equal to the half of the sum of higher
and lower limits of a class.
(ii) Assume the mean of any class-interval as assumed mean (A). But in doing so firstly remember
two things—first that assumed mean must be the mean of that class-interval whose frequency
is highest and second that assumed mean must be the mean of that class-interval which is
mean of given class-intervals.
(iii) find the difference (x – A)of the mean (x) of class-intervals and the assumed mean (A). in other
words calculate the difference (d) from the assumed mean of the mean of class intervals.
(iv) Thereafter find the sum (∑fd) of the multiplication (fd) of the frequency (f) with its related
deviation (d).
(v) find the result of the division of ((∑fd) and (∑fn) or add this result to the assumed mean (A).
(vi) this result will be arithmetic average.
if we present this technique in a formula this will be like-
S fd S f d
M = A + , or A +
N S f

loVely professional uniVersity 195