Page 150 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 7: Measures of Dispersion

From the above table Notes
510 2 356
X = 51 kgs and =35.6 kgs 2
10 10
= 5.97 kgs
2. Ungrouped or Grouped Frequency Distributions: Let the observations X , X ...... X appear
1 2 n
with respective frequencies f , f ...... f , where f N. As before, if the distribution is
1 2 n i
th
grouped, then X , X ...... X will denote the mid-values of the first, second ..... n class
1 2 n
intervals respectively. The formulae for the calculation of variance and standard deviation
can be written as
2 1 n 2 1 n 2
f X X f X X
i i and i i respectively.
N i 1 N i 1
Here also, we can show that
Variance = Mean of squares – Square of the mean
Therefore, we can write

f X 2 2 2 2
2 i i f X i and f X i f X i .
i
i
i
N N N N
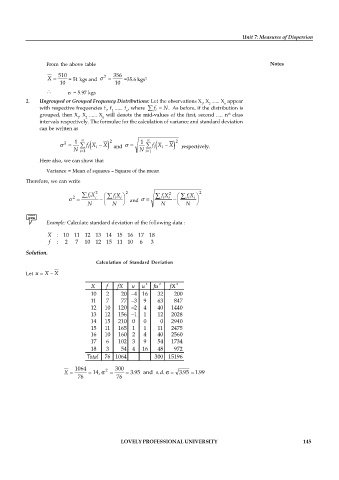
Example: Calculate standard deviation of the following data :

X : 10 11 12 13 14 15 16 17 18
f : 2 7 10 12 15 11 10 6 3

Solution.
Calculation of Standard Deviation
Let u X X

10 2 20 –4 16 32 200
11 7 77 –3 9 63 847
12 10 120 –2 4 40 1440
13 12 156 –1 1 12 2028
14 15 210 0 0 0 2940
15 11 165 1 1 11 2475
16 10 160 2 4 40 2560
17 6 102 3 9 54 1734
18 3 54 4 16 48 972
76 1064 300 15196
1064 2 300
.
14, 3 95 and . . 3 95 199
.
.
76 76

LOVELY PROFESSIONAL UNIVERSITY 145