Page 163 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 163
Quantitative Techniques – I
Notes Solution:
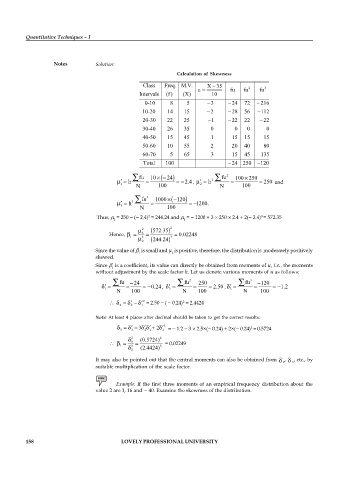
Calculation of Skewness
Class Freq. M.V. X 35 2 3
u fu fu fu
Intervals (f ) (X) 10
0-10 8 5 3 24 72 216
10-20 14 15 2 28 56 112
20-30 22 25 1 22 22 22
30-40 26 35 0 0 0 0
40-50 15 45 1 15 15 15
50-60 10 55 2 20 40 80
60-70 5 65 3 15 45 135
Total 100 24 250 120
fu 10 24 fu 2 100 250
h 2.4 , h 2 250 and
1 2
N 100 N 100
fu 3 1000 120
h 3 1200.
3
N 100
3
Thus, = 250 ( 2.4) = 244.24 and = 1200 + 3 250 2.4 + 2( 2.4) = 572.35
2
2 3
2 572.35 2
Hence, 3 0.02248
1 3 3
2 244.24
Since the value of is small and is positive, therefore, the distribution is moderately positively
1 3
skewed.
Since is a coefficient, its value can directly be obtained from moments of the moments
1
without adjustment by the scale factor . Let us denote various moments of as follows:
fu 24 fu 2 250 fu 3 120
1 0.24 , 2 2.50 , 3 1.2
N 100 N 100 N 100
2 = 2.50 ( 0.24) = 2.4424
2
2 2 1
Note: At least 4 places after decimal should be taken to get the correct results.
3 2 3 3
3 3 2 1 1 = 1.2 3 2.5×( 0.24) + 2×( 0.24) = 0.5724
2 2
3 (0.5724) = 0.02249
1 3 (2.4424) 3
2
It may also be pointed out that the central moments can also be obtained from , , etc., by
2 3
suitable multiplication of the scale factor.
Example: If the first three moments of an empirical frequency distribution about the
value 2 are 1, 16 and 40. Examine the skewness of the distribution.
158 LOVELY PROFESSIONAL UNIVERSITY