Page 159 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes
3.28 3.00
0.207
1.35
Since is positive and small, the distribution is moderately positively skewed.

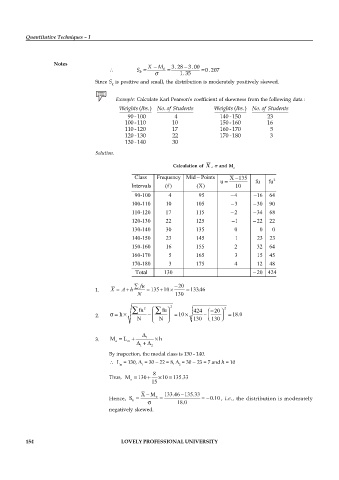
Example: Calculate Karl Pearson's coefficient of skewness from the following data :
Weights (lbs.) No. of Students Weights (lbs.) No. of Students
90 -100 4 140 -150 23
100 -110 10 150 -160 16
110 -120 17 160 -170 5
120 -130 22 170 -180 3
130 -140 30
Solution.

Calculation of X , and M
o
Class Frequency Mid Points X 135 2
u fu fu
Intervals (f ) (X) 10
90-100 4 95 4 16 64
100-110 10 105 3 30 90
110-120 17 115 2 34 68
120-130 22 125 1 22 22
130-140 30 135 0 0 0
140-150 23 145 1 23 23
150-160 16 155 2 32 64
160-170 5 165 3 15 45
170-180 3 175 4 12 48
Total 130 20 424

fu 20
.
1. X A h 135 10 13346
N 130
2 2
2
fu fu 424 20
2. h 10 18.0
N N 130 130

3. M o L m 1 h
1 2
By inspection, the modal class is 130 - 140.
= 130, = 30 22 = 8, = 30 23 = 7 and = 10
1 2
8
Thus, M 130 10 135.33
o
15
X M 133.46 135.33
Hence, S k o 0.10 , the distribution is moderately
18.0
negatively skewed.

154 LOVELY PROFESSIONAL UNIVERSITY