Page 304 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 15: Normal Probability Distribution

15.1 The Conditions of Normality Notes

In order that the distribution of a random variable X is normal, the factors affecting its observations
must satisfy the following conditions:

1. A large number of chance factors: The factors, affecting the observations of a random
variable, should be numerous and equally probable so that the occurrence or non-
occurrence of any one of them is not predictable.
2. Condition of homogeneity: The factors must be similar over the relevant population
although, their incidence may vary from observation to observation.
3. Condition of independence: The factors, affecting observations, must act independently of
each other.

4. Condition of symmetry: Various factors operate in such a way that the deviations of
observations above and below mean are balanced with regard to their magnitude as well
as their number.

15.2 Probability Density Function

If X is a continuous random variable, distributed normally with mean m and standard
2
1 X
1
deviation s, then its p.d.f. is given by p X .e 2 Where – < X < .
2
Here p and e are absolute constants with values 3.14159.... and 2.71828.... respectively.
It may be noted here that this distribution is completely known if the values of mean m and
standard deviation are known. Thus, the distribution has two parameters, viz. mean and
standard deviation.

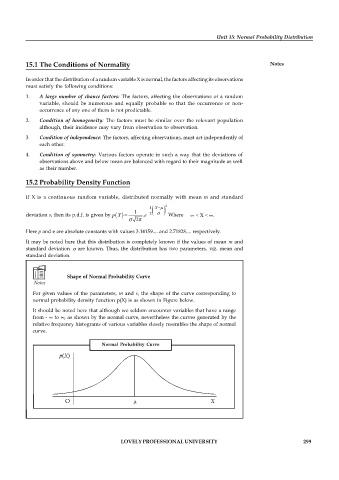
Shape of Normal Probability Curve
Notes
For given values of the parameters, m and s, the shape of the curve corresponding to
normal probability density function p(X) is as shown in Figure below.

It should be noted here that although we seldom encounter variables that have a range
from - to , as shown by the normal curve, nevertheless the curves generated by the
relative frequency histograms of various variables closely resembles the shape of normal
curve.

Normal Probability Curve

LOVELY PROFESSIONAL UNIVERSITY 299