Page 27 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

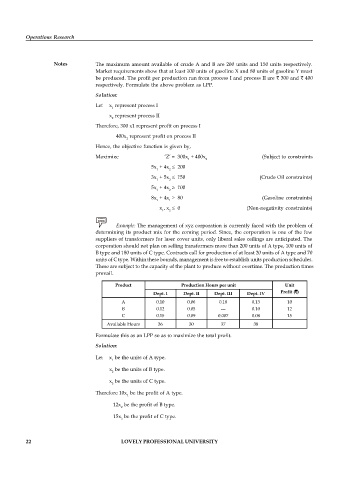
Notes The maximum amount available of crude A and B are 200 units and 150 units respectively.
Market requirements show that at least 100 units of gasoline X and 80 units of gasoline Y must
be produced. The profit per production run from process I and process II are ` 300 and ` 400
respectively. Formulate the above problem as LPP.
Solution:

Let x represent process I
1
x represent process II
2
Therefore, 300 x1 represent profit on process I
400x represent profit on process II
2
Hence, the objective function is given by,
Maximize ‘Z’ = 300x + 400x (Subject to constraints
1 2
5x + 4x  200
1 2
3x + 5x  150 (Crude Oil constraints)
1 2
5x + 4x  100
1 2
8x + 4x  80 (Gasoline constraints)
1 1
x , x  0 (Non-negativity constraints)
1 2

Example: The management of xyz corporation is currently faced with the problem of
determining its product mix for the coming period. Since, the corporation is one of the few
suppliers of transformers for laser cover units, only liberal sales ceilings are anticipated. The
corporation should not plan on selling transformers more than 200 units of A type, 100 units of
B type and 180 units of C type. Contracts call for production of at least 20 units of A type and 70
units of C type. Within these bounds, management is free to establish units production schedules.
These are subject to the capacity of the plant to produce without overtime. The production times
prevail.

Product Production Hours per unit Unit
Profit (`)
Dept. I Dept. II Dept. III Dept. IV
A 0.10 0.06 0.18 0.13 10
B 0.12 0.05 --- 0.10 12
C 0.15 0.09 0.007 0.08 15
Available Hours 36 30 37 38

Formulate this as an LPP so as to maximize the total profit.
Solution:

Let x be the units of A type.
1
x be the units of B type.
2
x be the units of C type.
3
Therefore 10x be the profit of A type.
1
12x be the profit of B type.
2
15x be the profit of C type.
3

22 LOVELY PROFESSIONAL UNIVERSITY