Page 117 - DECO405_MANAGERIAL_ECONOMICS

Managerial Economics

Notes The general production function is
Q = f (L, K)
If land, K, and labour, L, is multiplied by h and Q increases by , we get,
Q = f(hL, hK)
We have constant, increasing or decreasing returns to scale, respectively depending upon, whether
= h, > h or < h.
For example, if all inputs are doubled, we have constant, increasing or decreasing returns to
scale, respectively, if output doubles, more than doubles or less than doubles.
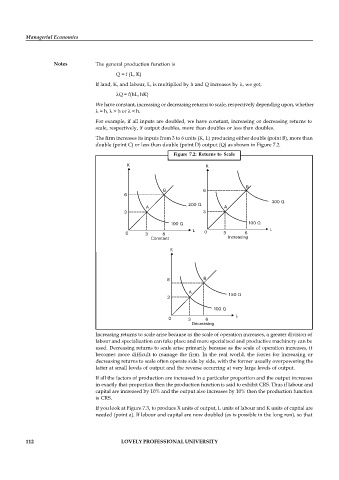
The firm increases its inputs from 3 to 6 units (K, L) producing either double (point B), more than
double (point C) or less than double (point D) output (Q) as shown in Figure 7.2.
Figure 7.2: Returns to Scale

Increasing returns to scale arise because as the scale of operation increases, a greater division of
labour and specialization can take place and more specialised and productive machinery can be
used. Decreasing returns to scale arise primarily because as the scale of operation increases, it
becomes more difficult to manage the firm. In the real world, the forces for increasing or
decreasing returns to scale often operate side by side, with the former usually overpowering the
latter at small levels of output and the reverse occurring at very large levels of output.
If all the factors of production are increased in a particular proportion and the output increases
in exactly that proportion then the production function is said to exhibit CRS. Thus if labour and
capital are increased by 10% and the output also increases by 10% then the production function
is CRS.
If you look at Figure 7.3, to produce X units of output, L units of labour and K units of capital are
needed (point a). If labour and capital are now doubled (as is possible in the long run), so that

112 LOVELY PROFESSIONAL UNIVERSITY