Page 149 - DECO405_MANAGERIAL_ECONOMICS

Managerial Economics

Notes so that

d TR d TC
= ... (3)
dQ dQ
Equation (3) indicates that in order to maximise profits, a firm produces where marginal
revenue (MR) equals marginal cost (MC). Since for a perfectly competitive firm, P is constant
and TR = (P).(Q) so that

d TR
= MR = P
dQ
the first order condition for profit maximisation for a perfectly competitive firm becomes
P = MR = MC.
The second order condition for profit maximisation requires that the second derivative of p with
respect to Q be negative. That is
2
2
d 2 d TR d TC
2 = 0 .... (4)
dQ dQ 2 dQ 2
2
2
d TR d TC
< .... (5)
dQ 2 dQ 2
According to equation (5) the algebraic value of the slope of the MC function must be greater
than the algebraic value of the MR function. Under perfect competition, MR is constant (MR
curve is horizontal). So that equation (5) requires that the MC curve be rising at the point where
MR=MC for the firm to maximise its total profits.

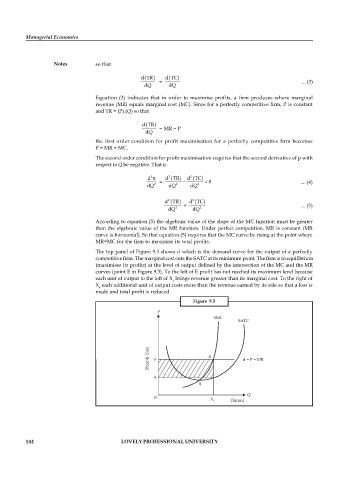
The top panel of Figure 9.3 shows d which is the demand curve for the output of a perfectly
competitive firm. The marginal cost cuts the SATC at its minimum point. The firm is in equilibrium
(maximises its profits) at the level of output defined by the intersection of the MC and the MR
curves (point E in Figure 9.3). To the left of E profit has not reached its maximum level because
each unit of output to the left of X brings revenue greater than its marginal cost. To the right of
e
X each additional unit of output costs more than the revenue earned by its sale so that a loss is
e
made and total profit is reduced.
Figure 9.3

144 LOVELY PROFESSIONAL UNIVERSITY