Page 51 - DECO405_MANAGERIAL_ECONOMICS

Managerial Economics

Notes 4.2 Law of Equi-marginal Utility

The Law of Equi-Marginal Utility is an extension to the law of diminishing marginal utility. The
principle of equi-marginal utility explains the behavior of a consumer in distributing his limited
income among various goods and services. This law states that how a consumer allocates his
money income between various goods so as to obtain maximum satisfaction.

Assumptions

The principle of equi-marginal utility is based on the following assumptions:

1. The wants of a consumer remain unchanged.
2. He has a fixed income.
3. The prices of all goods are given and known to a consumer.
4. He is one of the many buyers in the sense that he is powerless to alter the market price.
5. He can spend his income in small amounts.
6. He acts rationally in the sense that he want maximum satisfaction.

7. Utility is measured cardinally. This means that utility, or use of a good, can be expressed
in terms of "units" or "utils". This utility is not only comparable but also quantifiable.

Principle

Suppose there are two goods 'x' and 'y' on which the consumer has to spend his given income.
The consumer's behavior is based on two factors:
1. Marginal Utilities of goods 'x' and 'y'
2. The prices of goods 'x' and 'y'
The consumer is in equilibrium position when marginal utility of money expenditure on each
good is the same.
Mathematically, the law can be explained by the help of the following formula:
MU of good A/ Price of A = MU of good B/ Price of B
In any case when the Marginal Utilities of the goods A and B are unequal, the consumer will
purchase a combination that will give him highest Marginal Utility per dollar value of each
good, in such a way that the entire budget amount is spent.

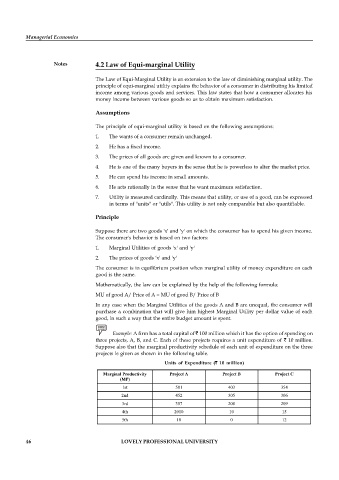
Example: A firm has a total capital of 100 million which it has the option of spending on
three projects, A, B, and C. Each of these projects requires a unit expenditure of 10 million.
Suppose also that the marginal productivity schedule of each unit of expenditure on the three
projects is given as shown in the following table.
Units of Expenditure ( 10 million)

Marginal Productivity Project A Project B Project C
(MP)
1st 501 403 354
2nd 452 305 306
3rd 357 208 209
4th 2010 10 15
5th 10 0 12

46 LOVELY PROFESSIONAL UNIVERSITY