Page 152 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

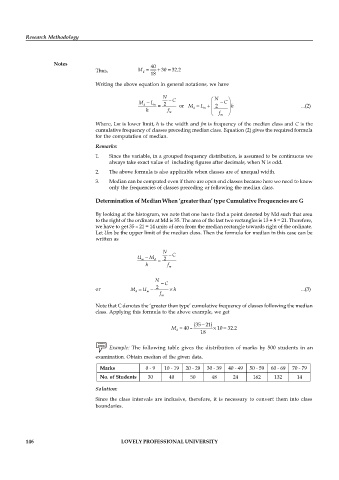
Notes 40
Thus, M   30  32.2
d
18
Writing the above equation in general notations, we have

N   N 
M  L m  2 C or M  L   2  C  ...(2)
d
h f m d m   f m   h

Where, Lm is lower limit, h is the width and fm is frequency of the median class and C is the
cumulative frequency of classes preceding median class. Equation (2) gives the required formula
for the computation of median.

Remarks:
1. Since the variable, in a grouped frequency distribution, is assumed to be continuous we
always take exact value of including figures after decimals, when N is odd.
2. The above formula is also applicable when classes are of unequal width.
3. Median can be computed even if there are open end classes because here we need to know
only the frequencies of classes preceding or following the median class.

Determination of Median When ‘greater than’ type Cumulative Frequencies are G
By looking at the histogram, we note that one has to find a point denoted by Md such that area
to the right of the ordinate at Md is 35. The area of the last two rectangles is 13 + 8 = 21. Therefore,
we have to get 35 – 21 = 14 units of area from the median rectangle towards right of the ordinate.
Let Um be the upper limit of the median class. Then the formula for median in this case can be
written as

N
U  M  C
m d  2
h f m

N
 C
or M  U  2  h ...(3)
d m
f
m
Note that C denotes the ‘greater than type’ cumulative frequency of classes following the median
class. Applying this formula to the above example, we get
35 21 
M  40 –  10  32.2
d
18

Example: The following table gives the distribution of marks by 500 students in an
examination. Obtain median of the given data.
Marks 0 - 9 10 - 19 20 - 29 30 - 39 40 - 49 50 - 59 60 - 69 70 - 79
No. of Students 30 40 50 48 24 162 132 14

Solution:
Since the class intervals are inclusive, therefore, it is necessary to convert them into class
boundaries.

146 LOVELY PROFESSIONAL UNIVERSITY