Page 153 - DMGT404 RESEARCH_METHODOLOGY

Unit 8: Descriptive Statistics

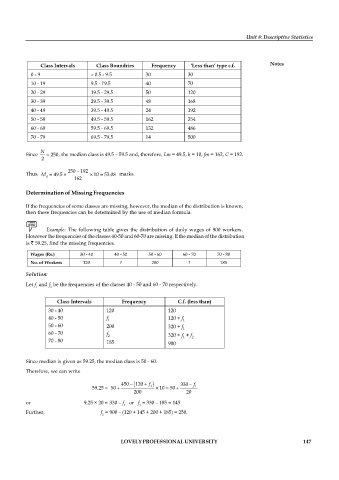
Class Intervals Class Boundries Frequency ‘Less than’ type c.f. Notes
0 - 9 – 0.5 - 9.5 30 30
10 - 19 9.5 - 19.5 40 70
20 - 29 19.5 - 29.5 50 120
30 - 39 29.5 - 39.5 48 168
40 - 49 39.5 - 49.5 24 192

50 - 59 49.5 - 59.5 162 354
60 - 69 59.5 - 69.5 132 486
70 - 79 69.5 - 79.5 14 500

N
Since  250, the median class is 49.5 – 59.5 and, therefore, Lm = 49.5, h = 10, fm = 162, C = 192.
2

Thus, M  49.5  250 192  10  53.08 marks.
d
162
Determination of Missing Frequencies

If the frequencies of some classes are missing, however, the median of the distribution is known,
then these frequencies can be determined by the use of median formula.

Example: The following table gives the distribution of daily wages of 900 workers.
However the frequencies of the classes 40-50 and 60-70 are missing. If the median of the distribution
is 59.25, find the missing frequencies.
Wages (Rs.) 30 - 40 40 - 50 50 - 60 60 - 70 70 - 80
No. of Workers 120 ? 200 ? 185

Solution:
Let f and f be the frequencies of the classes 40 - 50 and 60 - 70 respectively.
1 2

Class Intervals Frequency C.f. (less than)
30 - 40 120 120
40 - 50 f 120 + f
1
1
50 - 60 200 320 + f
1
60 - 70 f
2 320 + f + f
1
2
70 - 80 185 900

Since median is given as 59.25, the median class is 50 - 60.
Therefore, we can write
450  120  f  330 – f
59.25 = 50  1  10  50  1
200 20
or 9.25 × 20 = 330 – f or f = 330 – 185 = 145
1 1
Further, f = 900 – (120 + 145 + 200 + 185) = 250.
2

LOVELY PROFESSIONAL UNIVERSITY 147