Page 165 - DMGT404 RESEARCH_METHODOLOGY

Unit 8: Descriptive Statistics

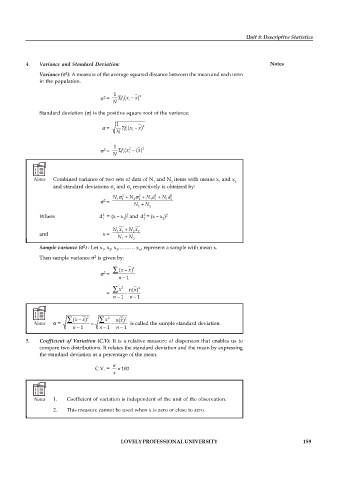
4. Variance and Standard Deviation: Notes
2
Variance ( ): A measure of the average squared distance between the mean and each term
in the population.
1 2
2
 = S i ( f x - ) x
i
N
Standard deviation () is the positive square root of the variance:
1 2
x
 = S i ( f x i – )
N
1 2 2
x
2
 = S i ( f x - ( )
i
N
Notes Combined variance of two sets of data of N and N items with means x and x
1 2 1 2
and standard deviations  and  respectively is obtained by:
1 2
N  + N  + N d + N d 2
2
2
2
2
2
 = 1 1 2 N + N 1 1 1 2
1 2
2
2
2
Where d = (x – x ) and d = (x – x ) 2
1 1 2 2
N x + N x
and x = 1 1 2 2
N + N 2
1
2
Sample variance ( ) : Let x , x , x ,……… x , represent a sample with mean x.
1 2 3 n
2
Then sample variance  is given by:
å ( – ) 2
x
x
2
 =
n - 1
å x 2 n(x) 2
= –
n - 1 n - 1
å (x x ) 2 å x 2 n ( ) 2
-
x
Notes  = = - is called the sample standard deviation.
n - 1 n - 1 n - 1
5. Coefficient of Variation (C.V): It is a relative measure of dispersion that enables us to
compare two distributions. It relates the standard deviation and the mean by expressing
the standard deviation as a percentage of the mean.

C.V. =  100
x

Notes 1. Coefficient of variation is independent of the unit of the observation.
2. This measure cannot be used when x is zero or close to zero.

LOVELY PROFESSIONAL UNIVERSITY 159