Page 243 - DMGT404 RESEARCH_METHODOLOGY

Unit 11: Index Numbers

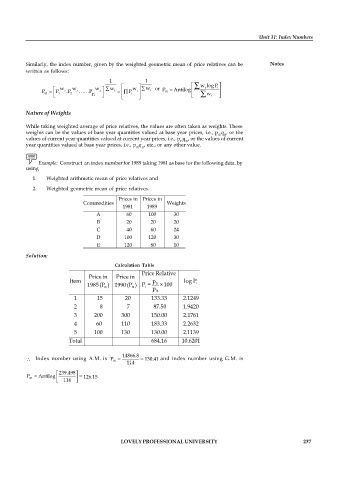
Similarly, the index number, given by the weighted geometric mean of price relatives can be Notes
written as follows:
1 1 é å w logP ù
ù
é
n å
P = é ê ë P 1 w 1 .P 2 w 2  P n w ù ú û w i = Õ P i w å w i or P = Antilogê ê ë å i w i i ú ú û
ê
ú
i
01
01
ú û
ê ë
Nature of Weights
While taking weighted average of price relatives, the values are often taken as weights. These
weights can be the values of base year quantities valued at base year prices, i.e., p q , or the
0i 0i
values of current year quantities valued at current year prices, i.e., p q , or the values of current
1i 1i
year quantities valued at base year prices, i.e., p q , etc., or any other value.
0i 1i
Example: Construct an index number for 1989 taking 1981 as base for the following data, by
using
1. Weighted arithmetic mean of price relatives and
2. Weighted geometric mean of price relatives.
Prices in Prices in
Commodities Weights
1981 1989
A 60 100 30
B 20 20 20
C 40 60 24
D 100 120 30
E 120 80 10

Solution:
Calculation Table
Price Relative
Price in Price in
Item p log P i
1985 (P ) 1990 (P ) P = 1i ×100
0i
0i
i
p
0i
1 15 20 133.33 2.1249
2 8 7 87.50 1.9420
3 200 300 150.00 2.1761
4 60 110 183.33 2.2632
5 100 130 130.00 2.1139
Total 684.16 10.6201

\ Index number using A.M. is P = 14866.8 = 130.41 and index number using G.M. is
01
114
P = Antilog é ê ë 239.498 ù ú = 126.15
114 û
01

LOVELY PROFESSIONAL UNIVERSITY 237