Page 246 - DMGT404 RESEARCH_METHODOLOGY
P. 246
Research Methodology
Notes å å å 0 å
1
1
´
1
1
P = P ´ P 01 Pa = å p q 0 0 ´ 100 ´ å p q 1 1 ´ 100 = å p q 0 å p q 1 1 ´ 100
La
F
01
01
p q
p q
p q
p q
0
0
0
0
å p q å p q
1
1
If we write L = å p q 0 0 and P = å p q 1 1 , the Fisher's Ideal Index can also be written as
0
0
P = L P ´ 100 .
´
01
4. Dorbish and Bowley's Index: This index number is constructed by taking the arithmetic
mean of the Laspeyres's and Paasche's indices.
1 é å p q å p q ù 1 é å p q 0 å p q ù
P 01 DB = ê 1 0 ´ 100 + 1 1 ´ 100ú = ê 1 + 1 1 ú ´ 100 = 1 [L × P] × 100
2 ê ëå p q 0 å p q 1 ú û 2 ê ëå p q 0 å p q 1 ú û 2
0
0
0
0
5. Marshall and Edgeworth's Index: This index number uses arithmetic mean of base and
current year quantities.
å p 1 ç æ è q + q 1 ö ÷ ø å p (q + q ) å p q + p q
0
0 å
P ME = 2 ´ 100 = 1 0 1 ´ 100 = 1 1 1 ´ 100
0 å
01 æ q + q 1 ö å p (q + q ) å p q + p q
å p 0 ç è 0 2 ÷ ø 0 0 1 0 0 1
6. Walsh's Index: Geometric mean of base and current year quantities are used as weights in
this index number.
Wa å p q q
0
P 01 = å p 1 0 q q 1 1 ´ 100
0
7. Kelly's Fixed Weights Aggregative Index: The weights, in this index number, are quantities
which may not necessarily relate to base or current year. The weights, once decided,
remain fixed for all periods. The main advantage of this index over Laspeyres's index is
that weights do not change with change of base year. Using symbols, the Kelly's Index can
be written as
Ke å p q
1
P 01 = å p q ´ 100
0
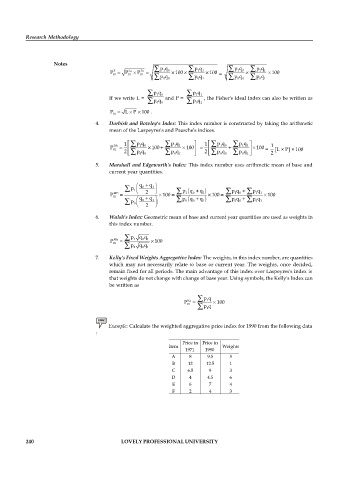
Example: Calculate the weighted aggregative price index for 1990 from the following data
:
Price in Price in
Item Weights
1971 1990
A 8 9.5 5
B 12 12.5 1
C 6.5 9 3
D 4 4.5 6
E 6 7 4
F 2 4 3
240 LOVELY PROFESSIONAL UNIVERSITY