Page 272 - DMGT404 RESEARCH_METHODOLOGY

Research Methodology

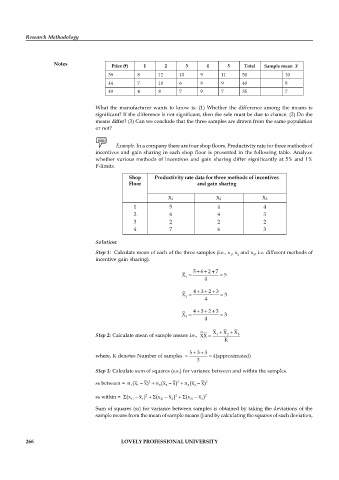
Notes Price ( ) 1 2 3 4 5 Total Sample mean x
39 8 12 10 9 11 50 10
44 7 10 6 8 9 40 8
49 4 8 7 9 7 35 7

What the manufacturer wants to know is: (1) Whether the difference among the means is
significant? If the difference is not significant, then the sale must be due to chance. (2) Do the
means differ? (3) Can we conclude that the three samples are drawn from the same population
or not?

Example: In a company there are four shop floors. Productivity rate for three methods of
incentives and gain sharing in each shop floor is presented in the following table. Analyze
whether various methods of incentives and gain sharing differ significantly at 5% and 1%
F-limits.
Shop Productivity rate data for three methods of incentives
Floor and gain sharing

X 1 X 2 X 3
1 5 4 4
2 6 4 3
3 2 2 2
4 7 6 3

Solution:
Step 1: Calculate mean of each of the three samples (i.e., x , x and x , i.e. different methods of
1 2 3
incentive gain sharing).
+
+
+
X = 5 6 2 7 = 5
1
4
+
+
+
4 3 2 3
X = = 3
2
4
+
+
+
X = 4 3 2 3 = 3
3
4
X + X + X
Step 2: Calculate mean of sample means i.e., XX = 1 2 3
K
+
+
where, K denotes Number of samples = 5 3 3 = 4(approximated)
3
Step 3: Calculate sum of squares (s.s.) for variance between and within the samples.
ss between = n (x - x) + n (x - x) + n (x - x) 2
2
2
2
2
1
1
3
3
ss within = (xS 1i - x ) + S (x - x ) + S (x - x ) 2
2
2
3i
1
3
2
2i
Sum of squares (ss) for variance between samples is obtained by taking the deviations of the
sample means from the mean of sample means () and by calculating the squares of such deviation,
266 LOVELY PROFESSIONAL UNIVERSITY